Suppose you have a sample of size n on three variables, y, x₁, and x₂, and you are primarily interested in the effect of x₁ on y. Let β̇₁ be the coefficient on x₁ from the simple regression and β̂₁ the coefficient on x₁ from the regression y on x₁, x₂. The standard errors reported by any regression package are

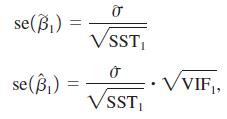
where s̆ is the SER from the simple regression, ŝ is the SER from the multiple regression, VIF₁ =  1/ 1 – R²₁), 2, and R²₁ is the R-squared from the regression of x₁ on x₂. Explain why se(β̂₁) can be smaller or larger than se(β̂|₁).

Transcribed Image Text:

se(B,) = VSST, se(ß,) = VVIF,, VSST,