A four-bar mechanism with ternary link is shown in Fig.3.9(a). The lengths of various links is given as below:

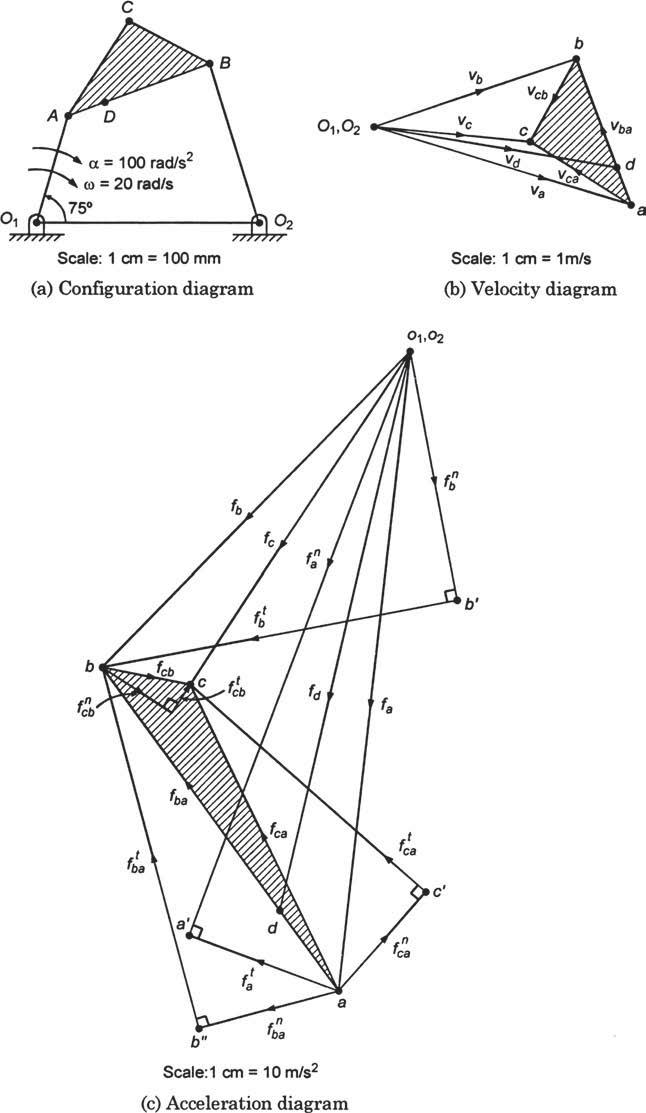\[ \begin{aligned} O_{1} O_{2} & =600 \mathrm{~mm}, O_{1} A=300 \mathrm{~mm}, A B=400 \mathrm{~mm}, O_{2} B=450 \mathrm{~mm} \\ A C & =300 \mathrm{~mm}, B C=250 \mathrm{~mm}, A D=100 \mathrm{~mm}, \text { and } \angle A O_{1} O_{2}=75^{\circ} \end{aligned} \]
Angular velocity of crank \(O_{1} A=20 \mathrm{rad} / \mathrm{s}\)
Angular acceleration of crank \(O_{1} A=100 \mathrm{rad} / \mathrm{s}^{2}\)
Determine

(a) acceleration of coupler \(A B\),

(b) acceleration of lever \(O_{2} B\),

(c) acceleration of points \(C\) and \(D\), and

(d) angular acceleration of ternary link.

C B A D a = 100 rad/s c = 20 rad/s 75 0 10. A b V Vcb Vba VC d 01,02 Vd Vca Va Scale: 1 cm 100 mm (a) Configuration diagram 01,02 Scale: 1 cm=1m/s (b) Velocity diagram fp fo 'b' b fcb fcb fa fba fpa fca 33 b" fba Scale: 1 cm = 10 m/s a (c) Acceleration diagram 8