Aircraft and space structures make use of plates and shells. The index depends on the configuration. Here
Question:
Aircraft and space structures make use of plates and shells. The index depends on the configuration. Here you are asked to derive the material index for
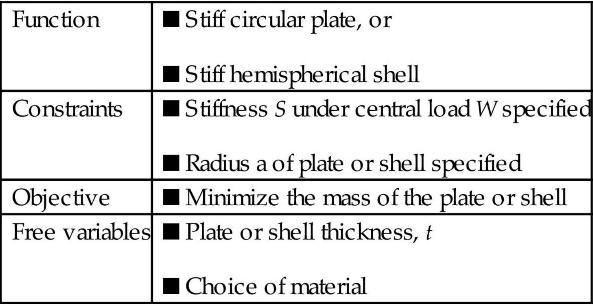
a. a circular plate of radius \(a\) carrying a central load \(W\) with a prescribed stiffness \(S=W / \delta\) and of minimum mass and
b. a hemispherical shell of radius \(a\) carrying a central load \(W\) with a prescribed stiffness \(S=W / \delta\) and of minimum mass, as shown in the figure.
\[\text { Circular plate: } \delta=\frac{3}{4 \pi} \frac{W a^{2}}{E t^{3}}\left(1-u^{2}\right)\left(\frac{3+u}{1+u}\right)\]
Hemispherical shell: \(\delta=A \frac{W a}{E t^{2}}\left(1-u^{2}\right)\)
in which \(A \approx 0.35\) is a constant. Here \(E\) is Young's modulus, \(t\) is the thickness of the plate or shell and \(u\) is Poisson's ratio. Poisson's ratio is almost the same for all structural materials and can be treated as a constant. The table included here summarizes the requirements.
Step by Step Answer:






