The bcc and diamond cubic structures of Table 12.1 are stable only when the bonds linking the

Question:

The bcc and diamond cubic structures of Table 12.1 are stable only when the bonds linking the atoms depend on direction. When bonding is nondirectional (when spheres are allowed to pack in the earths gravitational field, for example) packing can be loose-random, dense-random or close packed. Plot the packing fraction \(P\) against coordination number \(C\) for nondirectional packings. Is there a correlation?

Data From Table 12.1

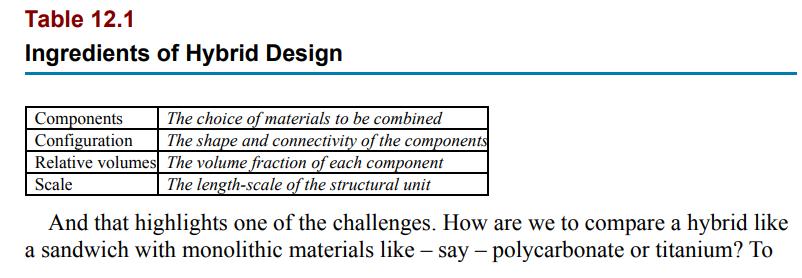
image text in transcribed

do this we must think of the sandwich not only as a hybrid with faces on one material bonded to a core of another, but as a ‘material’ in its own right, with its own set of ‘effective properties’; it is these that allow the comparison. The approach adopted here is one of breadth rather than precision. The aim is to assemble methods to allow the properties of alternative hybrids to be scanned and compared, seeking those that best meet a given set of design requirements. Once materials and configuration have been chosen, standard methods – optimization routines, finite-element analyses – can be used to refine them. But what the standard methods are not good at is the quick scan of alternative combinations. That is where the approximate methods developed below, in which material and configuration become the variables, pay off. The word ‘configuration’ requires elaboration. four different configurations of bridge. In the first all members are loaded in compression. In the second, members carry both tension and compression, depending on how the bridge is loaded. In the third and fourth, the suspension cables are loaded purely in tension. Any one of these can be optimized, but no amount of optimization will cause one to evolve into another because this involves a discrete jump in configuration, each characterized by its own set of variables.