Kimball Company has developed the following cost formulas: The company has a policy of producing on demand
Question:
Kimball Company has developed the following cost formulas:
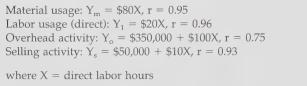
The company has a policy of producing on demand and keeps very little, if any, finished goods inventory (thus, units produced = units sold).
The president of Kimball Company has recently implemented a policy that any special orders will be accepted if they cover the costs that the orders cause. This policy was implemented because Kimball’s industry is in a recession and the company is producing well below capacity (and expects to continue doing so for the coming year). The president is willing to accept orders that at least cover their variable costs so that the company can keep its employees and avoid layoffs. Also, any orders above variable costs will increase overall profitability of the company.
Required:
1. Compute the total unit variable cost. Suppose that Kimball has an opportunity to accept an order for 20,000 units at $220 per unit. Each unit uses one direct labor hour for production. Should Kimball accept the order? (The order would not displace any of Kimball’s regular orders.)
2. Explain the significance of the coefficient of correlation measures for the cost formulas. Did these measures have a bearing on your answer in requirement 1?
Should they have a bearing? Why?
3. Suppose that a multiple regression equation is developed for overhead costs: Y = $100,000 + $100X, + $5,000X, + $300X;3, where X, = direct labor hours, X, = number of setups, and X; = engineering hours. The correlation coefficient for the equation is 0.94. Assume that the order of 20,000 units requires 12 setups and 600 engineering hours. Given this new information, should the company accept the special order referred to in Requirement 1? Is there any other information about cost behavior that you would like to have? Explain.lop5
Step by Step Answer:






