The Hamiltonian operator for a one-dimensional harmonic oscillator moving in the x direction is Find the value
Question:
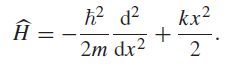
Find the value of the constant a such that the function eˆ’ax2 is an eigenfunction of the Hamiltonian operator and find the eigenvalue E. The quantity k is the force constant, m is the mass of the oscillating panicle, and „ is Planck€™s constant divided by 2Ï€.
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Divide by e ax2 The coefficients of x 2 on the two sides o...View the full answer
Answered By
Utsab mitra
I have the expertise to deliver these subjects to college and higher-level students. The services would involve only solving assignments, homework help, and others.
I have experience in delivering these subjects for the last 6 years on a freelancing basis in different companies around the globe. I am CMA certified and CGMA UK. I have professional experience of 18 years in the industry involved in the manufacturing company and IT implementation experience of over 12 years.
I have delivered this help to students effortlessly, which is essential to give the students a good grade in their studies.
2+ Reviews
10+ Question Solved
Related Book For 
Question Posted:
