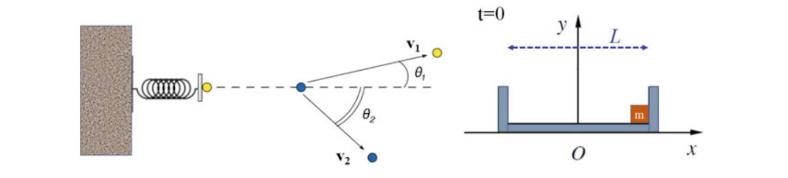
A small ball of mass \(M=800 \mathrm{~g}\) and negligible size lies on a smooth horizontal plane and is initially attached to the end of a spring of spring constant \(k\) so as to compress it by a distance \(d_{0}=2.00 \mathrm{~cm}\). The ball is subsequently released and moves across the plane until it collides with a stationary identical ball. The two balls emerge from the collision respectively with speed \(v_{1}=6.2\) \(\mathrm{m} / \mathrm{s}\) and \(v_{2}=3.3 \mathrm{~m} / \mathrm{s}\) at angles \(\theta_{1}\) and \(\theta_{2}\) with respect to the initial direction of the incident ball, as shown in Fig. 8.14. Determine:

1. the angle \(\theta_{2}\), knowing that \(\theta_{1}=18.5^{\circ}\);

2. the linear momentum of the two ball system after the collision;

3 . the value of the elastic constant \(k\) of the spring;

4. whether the collision is elastic or inelastic. In the case where the collision is inelastic, calculate the energy dissipated.

5. Prove that in the case of elastic impact there is: \(\theta_{1}+\theta_{2}=90^{\circ}\).

Fig. 8.14

Transcribed Image Text:

t=0 y 82 0 m