Now consider overdispersion in the Poisson model. (a) Suppose (Y mid mu sim mathcal{P}[mu]), where (mu=exp left(beta_{0}+beta_{1}
Question:
Now consider overdispersion in the Poisson model.
(a) Suppose \(Y \mid \mu \sim \mathcal{P}[\mu]\), where \(\mu=\exp \left(\beta_{0}+\beta_{1} x\right), \beta_{0}=\gamma_{0}+\varepsilon\), and \(\varepsilon\) is an unobserved random variable with \(\mathrm{E}[\varepsilon]=0, \mathrm{~V}[\varepsilon]=\sigma^{2}>0\). Show that \(V[Y]>E[Y]\).
(b) Consider the NB2 model with the variance function \(\mu+\alpha \mu^{2}\) and the probability mass function given in (20.12). Using graphs for four different values of \(\alpha \in[0,3]\), describe the behavior of the probability mass for different realized values of \(Y\); in your answer concentrate on the behavior of the function near the origin and in the right tail.
(c) For the NB2 density given in (20.12) in Section 20.4.1, show that as \(\alpha \rightarrow 0\) the density goes to the Poisson. [This could be tricky.]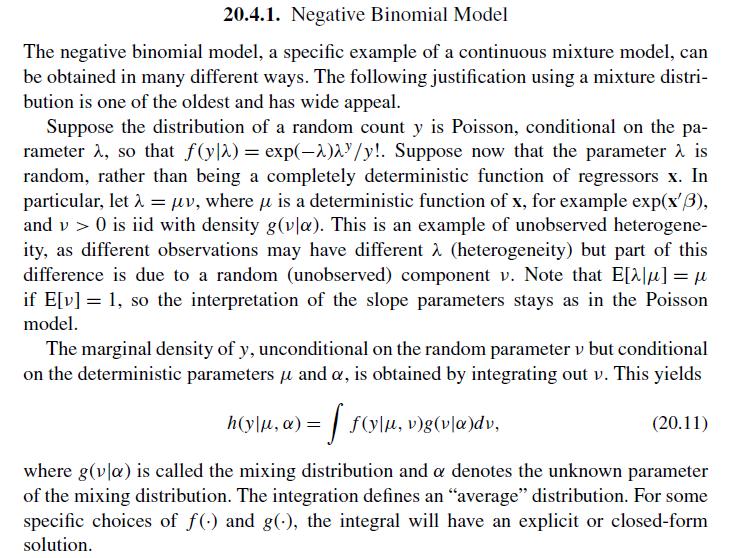
Step by Step Answer:

Microeconometrics Methods And Applications
ISBN: 9780521848053
1st Edition
Authors: A.Colin Cameron, Pravin K. Trivedi





