(a) By taking the trace of the time-dependent tensorial virial theorem and specializing to an MHD plasma...
Question:
(a) By taking the trace of the time-dependent tensorial virial theorem and specializing to an MHD plasma with (or without) self-gravity, show that
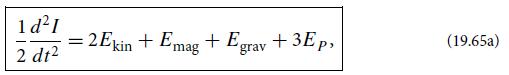
where I is the trace of Ijk, Ekin is the system’s kinetic energy, Emag is its magnetic energy, EP is the volume integral of its pressure, and Egrav is its gravitational self-energy:
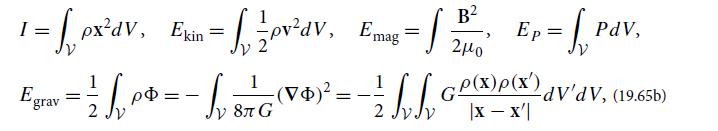
with Φ the gravitational potential energy [cf. Eq. (13.62)].
(b) When the time integral of d2I/dt2 vanishes, then the time average of the righthand side of Eq. (19.65a) vanishes:
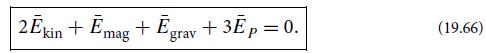
This is the time-averaged scalar virial theorem. Give examples of circumstances in which it holds.
(c) Equation (19.66) is a continuum analog of the better-known scalar virial theorem, 2E̅kin + E̅grav = 0, for a system consisting of particles that interact via their self-gravity—for example, the solar system.
(d) As a simple but important application of the time-averaged scalar virial theorem, show—neglecting self-gravity—that it is impossible for internal currents in a plasma to produce a magnetic field that confines the plasma to a finite volume: external currents (e.g., in solenoids) are necessary.
(e) For applications to the oscillation and stability of self-gravitating systems.
Equation 13.62.
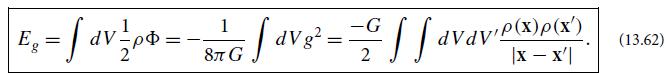
Step by Step Answer:

Modern Classical Physics Optics Fluids Plasmas Elasticity Relativity And Statistical Physics
ISBN: 9780691159027
1st Edition
Authors: Kip S. Thorne, Roger D. Blandford





