Consider a knife on its back, so its sharp edge points in the upward, z direction. The
Question:
Consider a knife on its back, so its sharp edge points in the upward, z direction. The edge (idealized as extending infinitely far in the y direction) is hot, and by heating adjacent fluid, it creates a rising thermal plume. Introduce a temperature deficit △T (z) that measures the typical difference in temperature between the plume and the surrounding, ambient fluid at height z above the knife edge, and let δp(z) be the width of the plume at height z.
(a) Show that energy conservation implies the constancy of δp△T v̅z, where v̅z(z) is the plume’s mean vertical speed at height z.
(b) Make an estimate of the buoyancy acceleration, and use it to estimate v̅z.
(c) Use Eq. (18.19) to relate the width of the plume to the speed. Hence, show that the width of the plume scales as δp ∝ z2/5 and the temperature deficit as △T ∝ z−3/5.
(d) Repeat this exercise for a 3-dimensional plume above a hot spot.
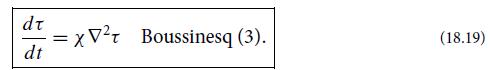
Step by Step Answer:
a To show that energy conservation implies the constancy of pT vz we can consider the energy balance of the rising thermal plume The energy transported by the plume per unit time can be expressed as t...View the full answer
Modern Classical Physics Optics Fluids Plasmas Elasticity Relativity And Statistical Physics
ISBN: 9780691159027
1st Edition
Authors: Kip S. Thorne, Roger D. Blandford
