A beam of weight W per unit length is simply supported at the same level at (N + 1) equidistant points, the extreme supports being at the ends of the beam. The bending moment M_k at the kth support satisfies the recurrence relation

where a is the distance between the supports and M₀ = 0 and M_N = 0. (This is a consequence of Clapeyron’s theorem of three moments.) Show that if the sequences

are calculated by the recurrences

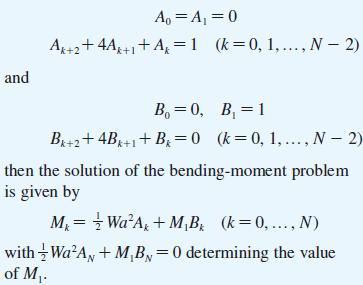
Perform the calculation for the case where N = 8, a = 1 and W = 25.

Transcribed Image Text:

M+2+4Mk+1 + M = = Wa