In this application from major league baseball, the populations represent an abstraction of what the players can

Question:

In this application from major league baseball, the populations represent an abstraction of what the players can do, so the populations will vary from year to year. The Colorado Rockies and the Arizona Diamondbacks played nine games in Phoenix and ten games in Denver in 2001. The The thinner air in Denver causes curve balls to curve less and it allows fly balls to travel farther. Does this mean that more runs are scored in Denver? The numbers of runs scored by the two teams in the nine Phoenix games (P) and ten Denver games (D) are

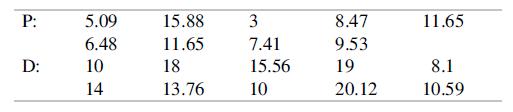
The fractions occur because the numbers have been adjusted for nine innings (54 outs). For example, in the third Denver game the Rockies won 10 to 7 on a home run with two out in the bottom of the tenth inning, so there were 59 outs instead of 54, and the number of runs is adjusted to (54/59)(17) = 15.56. We want to compare the average runs in Denver with the average runs in Phoenix.
a. Find a 95% confidence interval for the difference of population means using the two-sample t interval.
b. Use software to generate a bootstrap sample of differences of means. Check the bootstrap distribution for normality using a normal probability plot.
c. Use the standard deviation of the bootstrap distribution along with the mean and t critical value from (a) to get a 95% confidence interval for the difference of means.
d. Use the bootstrap sample and the percentile method to obtain a 95% confidence interval for the difference of means.
e. Compare your three confidence intervals. If you used a standard normal critical value in place of the t critical value in (c), why would that make this interval more like the one in (d)? Why should the three intervals be fairly similar for this data set?
f. Interpret your results. Is there a substantial difference between the two locations? Compare the difference with what you thought it would be. If you were a major league pitcher, would you want to be traded to the Rockies?