Demonstrate that the identity, reflections about the three symmetry axes (dashed lines), and rotations by \(\frac{2 \pi}{3}\) and \(\frac{4 \pi}{3}\) about the center of the equilateral triangle

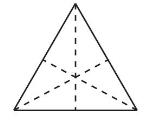
form a group of order six (the dihedral group, \(\mathrm{D}_{3}\) ) that is isomorphic to the permutation group \(\mathrm{S}_{3}\). Show that \(\mathrm{D}_{3}\) has four distinct subgroups. (This result is a special case of Cayley's theorem: every group of order \(n\) is isomorphic to a subgroup of \(\mathrm{S}_{n}\).)

Transcribed Image Text:

A