If the assumptions leading to the Bose-Einstein distribution are modified so that the number of particles is
Question:
If the assumptions leading to the Bose-Einstein distribution are modified so that the number of particles is not assumed constant, the resulting distribution has ea = 1. This distribution can be applied to a “gas” of photons. Consider the photons to be in a cubic box of side L. The momentum components of a photon are quantized by the standing-wave conditions kx = n1π/L, ky = n2π/L, ky = n2π/L, and kz = n3π/L, where p = ћ (k2x + k2y + k2z)1/2 is the magnitude of the momentum.
(a) Show that the energy of a photon can be written E = N(ћcπ/L), where N2 = n21 + n22 + n23.
(b) Assuming two photons per space state because of the two possible polarizations, show that the number of states between N and N + dN is πN2 dN.
(c) Find the density of states and show that the number of photons in the energy interval dE is
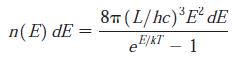
(d) The energy density in dE is given by u(E) dE = En(E) dE/L3. Use this to obtain the Planck blackbody radiation formula for the energy density in dλ, where λ is the wavelength:
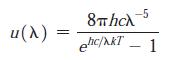
Step by Step Answer:






