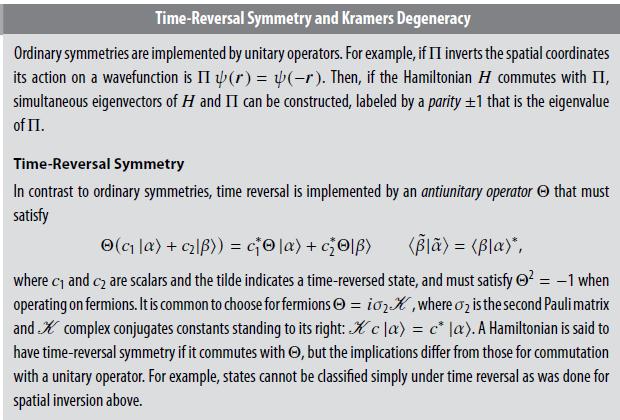
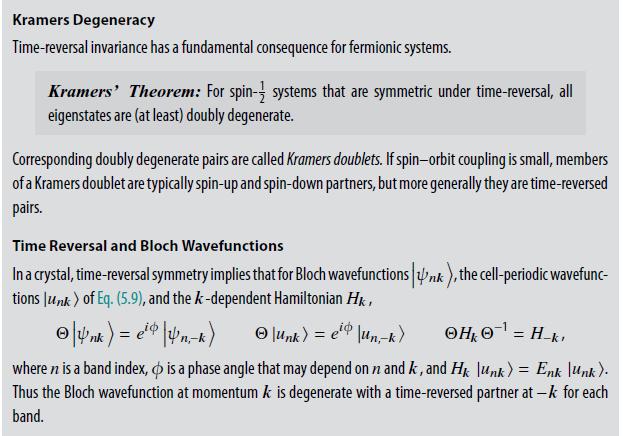
Prove Kramers' theorem (Box 29.1 ) for spin- $\frac{1}{2}$ fermions by showing that a state and its time reverse are degenerate and orthogonal, so they are two independent states with the same energy. Use that the time-reversal operator obeys $\Theta^{2}=-1$, and that $\langle\tilde{\beta} \mid \tilde{\alpha}angle=\langle\beta \mid \alphaangle^{*}$ from Problem 29.4 .

Data from Box 29.1

Data from Problem 29.4

For states $|\alphaangle$ and $|\betaangle$, define time-reversed states by $|\tilde{\alpha}angle=\Theta|\alphaangle$ and $|\tilde{\beta}angle=\Theta|\betaangle$. Show that the overlap of the time-reversed states is given by $\langle\tilde{\beta} \mid \tilde{\alpha}angle=\langle\beta \mid \alphaangle^{*}$. Expand $|\tilde{\alpha}angle$ and $|\tilde{\beta}angle$ in complete sets of states using $\sum_{a}|aangle\langle a|=1$, and use that the complex conjugation operator has no effect on a ket, $\mathscr{K}|\alphaangle=|\alphaangle$.

Transcribed Image Text:

Time-Reversal Symmetry and Kramers Degeneracy Ordinary symmetries are implemented by unitary operators. For example, if II inverts the spatial coordinates its action on a wavefunction is II (r) = (-). Then, if the Hamiltonian H commutes with II, simultaneous eigenvectors of H and II can be constructed, labeled by a parity 1 that is the eigenvalue of II. Time-Reversal Symmetry In contrast to ordinary symmetries, time reversal is implemented by an antiunitary operator that must satisfy (ca) cl)) = cola) + co|)