Show that for a continuous parameter (theta) the set of matrices forms a one-parameter abelian Lie group

Question:

Show that for a continuous parameter \(\theta\) the set of matrices

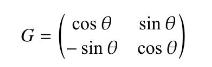
image text in transcribed

forms a one-parameter abelian Lie group under matrix multiplication, and that if the matrices \(G\) operate on a 2D cartesian space \(\left(x_{1}, x_{2}\right)\) they leave \(x_{1}^{2}+x_{2}^{2}\) invariant.

Fantastic news! We've Found the answer you've been seeking!

Step by Step Answer:

Related Book For answer-question

Symmetry Broken Symmetry And Topology In Modern Physics A First Course

ISBN: 9781316518618

1st Edition

Authors: Mike Guidry, Yang Sun

See More Books

Question Posted: Mar 27, 2024 06:01 AM

See More Questions

Portray in words what transforms you would have to make to your execution to some degree (a) to accomplish this and remark on the benefits and detriments of this thought.You are approached to compose...
"internet radios" for streaming audio, and personal video recorders and players. Describe design and evaluation processes that could be used by a start-up company to improve the usability of such...
Briefly describe ASCII and Unicode and draw attention to any relationship between them. [3 marks] (b) Briefly explain what a Reader is in the context of reading characters from data. [3 marks] A...
Problem Set 3 b. zero. c. negative. d. smaller than the variance. 22. Growth factors for the population of Atlanta in the past five years have been 1, 2, 3, 4, and 5. The geometric mean is a. 15. b....
The financial statements and accompanying notes of Corus Entertainment are presented in Appendix A at the end of this book. Instructions (a) What classifications does Corus use in its balance sheet?...
Selected accounts from the chart of accounts of Valdez Company are shown below. 101 ...........Cash 112 ...........Accounts Receivable 120 ...........Inventory 126 ...........Supplies 157...
If a well-behaved investment alternative's internal rate of return (IRR) is equal to MARR, which of the following statements about the other measures of worth for this alternative must be true? 1....
On June 1, 2014, Ellie Hopkins established an interior decorating business, First-Class Designs. During the month, Ellie completed the following transactions related to the business: June 1. Ellie...
Compare and contrast online transaction processing (OLTP) and online analytical processing (OLAP) databases.
Show that the Pauli matrices generate a matrix representation of Lie group elements having the form as required by Problem 3.4 . Data from Problem 3.4 Show that the most general form for \(2 \times...
Matrices are central to representation theory. If \(A, B, C\), and \(D\) are square, invertible, \(n \times n\) matrices with complex entries, prove the following useful properties. a. The trace of a...
Continuation of Exercise 4-135. With an automated irrigation system, a plant grows to a height of 3.5 centimeters two weeks after germination. (a) What is the probability of obtaining a plant of this...
Are there alternatives to the repealed individual mandate that accomplish the same goals without engendering so much political turmoil? Could policymakers have designed an incentive system that would...
Mention at least 4 scales from the Meyer book Discuss what each scale means and cite from the book using APA, . Give concrete examples of how these scales work in your culture. Contrast your own...
A nurse is admitting a client with suspected bacterial meningitis. What are three (3) interventions that the nurse should include in the plan of care?
Explain the statement and give concrete examples, "Words don't mean; people do.".
How do you resolve a DNA-related problem caused by an invalid DNS cache entry ?
Repeat Prob. 10.75, to find the time to drain the tank from 1.0 m to 50 cm, if the gate is drowned downstream at y2 = 40 cm. Again assume gap H = 10 cm.
Problem 3.5 (4 points). We will prove, in steps, that rank (L) = rank(LT) for any LE Rnxm (a) Prove that rank (L) = rank (LTL). (Hint: use Problem 3.4.) (b) Use part (a) to deduce that that rank(L) =...
A secured creditor will usually include terms in the security agreement with a debtor giving the creditor the right, in the event of a default by the debtor, to Multiple choice question. take over...
In this problem we will perform simple regression according to the CAPM model. An incomplete code is provided in hw2.r . First, read hw2.r to nd out how to use package "quantmod" to get historical...
When a client hires an attorney to represent them; under this arrangement, the lawyer keeps a percentage of the money recovered on a client's behalf. If there is no recovery for the client, the...

Show that for a continuous parameter (theta) the set of matrices forms a one-parameter abelian Lie group

Question:

cos e G = sin 0 sin 0 cos 0)

Step by Step Answer:

The produc...View the full answer

Symmetry Broken Symmetry And Topology In Modern Physics A First Course

Students also viewed these Sciences questions