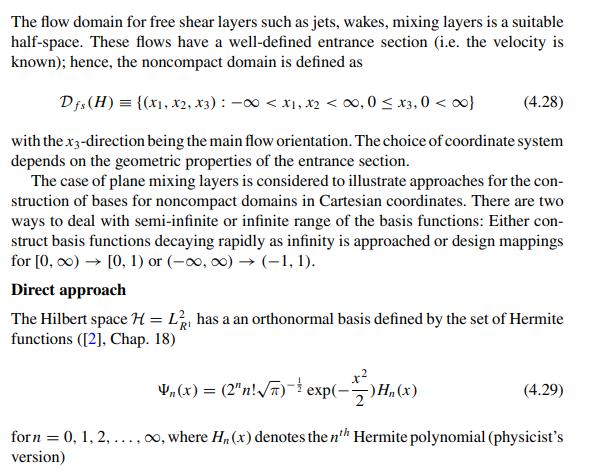
The Hermite functions (Sect. 4.3.3) are a basis for the space \(\Omega=\) \(C_{R^{1}}^{\infty} \cap L_{R^{1}}^{2}\) of functions defined on the unbounded domain \(R^{1}\). Determine the coordinates \(c_{i}\) with respect to the basis \(\left\{\Psi_{n}(x): n=0,1, \ldots, \infty\right\}\) of the test function

\[ f(x)=\frac{\cos (k x)}{\sqrt{2 \pi \sigma^{2}}} \exp \left(-\frac{1}{2 \sigma^{2}}\left(x-x_{0}\right)^{2}\right) \]

for \(k=4, x_{0}=0.0\) and \(\sigma=0.5\) as defined in Eq. (4.33) of Sect. 4.3.3. Compute the maximum norm of the error

\[ e(N) \equiv\left|f(x)-\sum_{i=0}^{N} c_{i} \Psi_{i}^{x}\right| \]

as function of \(N\). Plot the coefficients \(c_{i}\), the error \(e(N)\) and the test function compared to the approximation for \(N=20\) terms.

Sect. 4.3.3

Eq. (4.33)

Transcribed Image Text:

The flow domain for free shear layers such as jets, wakes, mixing layers is a suitable half-space. These flows have a well-defined entrance section (i.e. the velocity is known); hence, the noncompact domain is defined as Dfs (H) = {(x1, x2, x3): -00 < x1, x2 < 00, 0 x3, 0 < 0} (4.28) with the x3-direction being the main flow orientation. The choice of coordinate system depends on the geometric properties of the entrance section. The case of plane mixing layers is considered to illustrate approaches for the con- struction of bases for noncompact domains in Cartesian coordinates. There are two ways to deal with semi-infinite or infinite range of the basis functions: Either con- struct basis functions decaying rapidly as infinity is approached or design mappings for [0, ) [0, 1) or (-, ) (-1, 1). Direct approach R The Hilbert space H = L has a an orthonormal basis defined by the set of Hermite functions ([2], Chap. 18) Vn(x)=(2"n!) exp(- -)Hn(x) (4.29) forn = 0, 1, 2,..., 00, where H, (x) denotes the nth Hermite polynomial (physicist's version)