32 Ballco manufactures large softballs, regular softballs, and hardballs. Each type of ball requires time in three departments: cutting, sewing, and packaging, as shown in Table 65 (in minutes). Because of marketing considerations, at least 1,000 regular softballs must be produced. Each

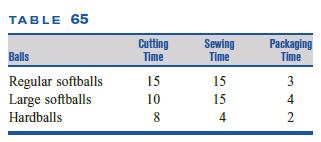
regular softball can be sold for $3, each large softball, for $5; and each hardball, for $4. A total of 18,000 minutes of cutting time, 18,000 minutes of sewing time, and 9,000 minutes of packaging time are available. Ballco wants to maximize sales revenue. If we define RS number of regular softballs produced LS number of large softballs produced HB number of hardballs produced then the appropriate LP is max z 3RS 5LS 4HB s.t. 15RS 10LS 8HB 18,000 s.t. 15RS 15LS 4HB 18,000 s.t. 3RS 4LS 2HB 9,000 s.t. RS 15LS 2HB 1,000 RS, LS, HB 0 The optimal tableau for this LP is shown in Table 66.
a Find the dual of the Ballco problem and its optimal solution.
b Show that the Ballco problem has an alternative optimal solution. Find it. How many minutes of sewing time are used by the alternative optimal solution?
c By how much would an increase of 1 minute in the amount of available sewing time increase Ballco’s revenue?
How can this answer be reconciled with the fact that the sewing constraint is binding? (Hint: Look at the answer to part (b).)
d Assuming the current basis remains optimal, how would an increase of 100 in the regular softball requirement affect Ballco’s revenue?

Transcribed Image Text:

TABLE 65 Cutting Sewing Balls Time Time Packaging Time Regular softballs 15 Large softballs Hardballs 108 15 15 4 342 3 4 2