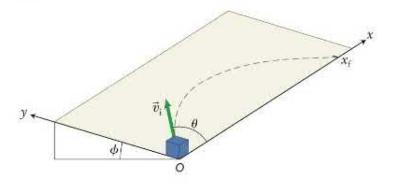
A block of inertia \(m\) sits at the origin of an \(x y\) coordinate system aligned along an incline that makes an angle \(\phi\) with respect to the horizontal (Figure P10.57). You launch the block up the incline, with initial speed \(v_{\mathrm{i}}\) directed at an angle \(\theta\) with respect to the \(x\) axis in the plane of the incline.

(a) What are the magnitude and direction of the block's acceleration expressed in terms of the indicated coordinate system?

(b) Derive an expression for the block's velocity as a function of time, expressed in unit vector notation for the indicated coordinate system.

(c) What is the maximum value of the block's displacement \(\Delta y\) along the \(y\) axis?

(d) What is the block's maximum displacement \(\Delta x\) along the \(x\) axis? Ignore friction.

Data from Figure P10.57