A solid sphere of radius \(R\) carries a fixed, uniformly distributed charge \(q\). Exploiting the analogy between Newton's law of gravity and Coulomb's law, use the result obtained in Section 13.8 to obtain an expression for the magnitude of the electric field created by the sphere at a point \(P\) outside the sphere.

Data from Section 13.8

Transcribed Image Text:

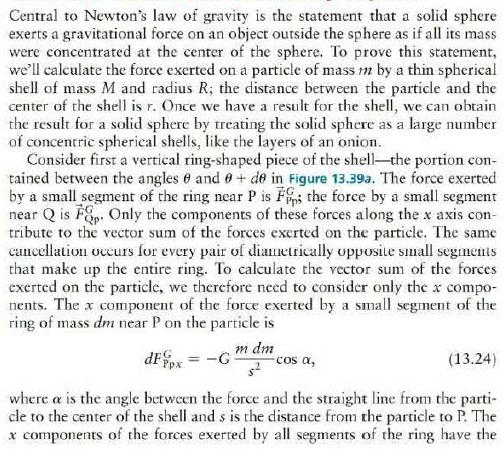
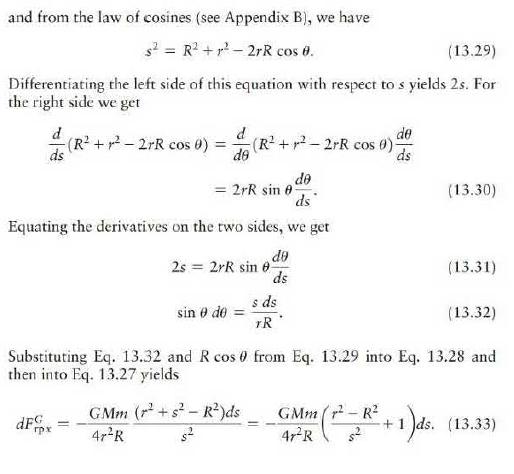
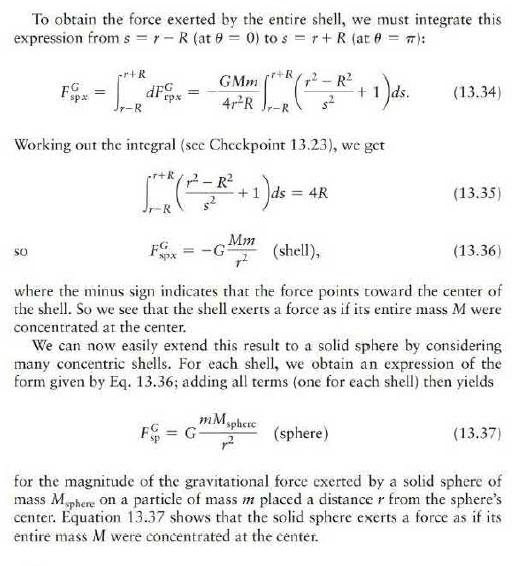
Central to Newton's law of gravity is the statement that a solid sphere exerts a gravitational force on an object outside the sphere as if all its mass were concentrated at the center of the sphere. To prove this statement, we'll calculate the force exerted on a particle of mass by a thin spherical shell of mass M and radius R; the distance between the particle and the center of the shell is r. Once we have a result for the shell, we can obtain the result for a solid sphere by treating the solid sphere as a large number of concentric spherical shells, like the layers of an onion. Consider first a vertical ring-shaped piece of the shell-the portion con- tained between the angles 0 and 0+de in Figure 13.39a. The force exerted by a small segment of the ring near P is F; the force by a small segment near Q is F. Only the components of these forces along the x axis con- tribute to the vector sum of the forces exerted on the particle. The same cancellation occurs for every pair of diametrically opposite small segments that make up the entire ring. To calculate the vector sum of the forces exerted on the particle, we therefore need to consider only the x compo- nents. The x component of the force exerted by a small segment of the ring of mass dm near P on the particle is m dm dFx=-G cos , (13.24) where a is the angle between the force and the straight line from the parti- cle to the center of the shell and s is the distance from the particle to P. The x components of the forces exerted by all segments of the ring have the