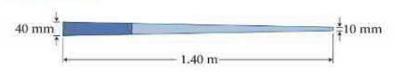
Determine the center of mass of a pool cue whose diameter decreases smoothly from \(40 \mathrm{~mm}\) to \(10 \mathrm{~mm}\) over its \(1.40-\mathrm{m}\) length (Figure P6.44). Assume that the cue is made from solid wood, with no hidden weights inside, (Hint: See Appendix D for the center-of-mass computation for extended objects. You will find it easier to do the integral for a complete cone. The pool cue is a truncated cone-that is, a cone with its conical tip removed. Slicing off a piece is like adding negative inertia.)

Data from Figure P6.44

Transcribed Image Text:

40 mm 1.40 m- 10 mm