For a free particle of momentum p = (p_x, p_y, p_z), the Dirac equation has four independent solutions (1.12), where the independent spinors can be chosen to be of the forms

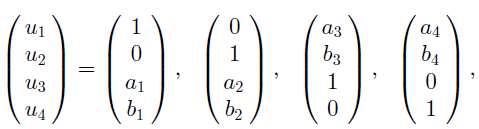where a_i, b_i are finite coefficients, if we assume the explicit form of the Dirac matrices given in Problem 1.2. Verify that solutions of these forms exist and find the values of the coefficients a_i, b_i and the corresponding energies.

Data From Problem 1.2

The matrices α_i (i = 1, 2, 3) and β in the Dirac equation (1.8) can be chosen to have the explicit forms

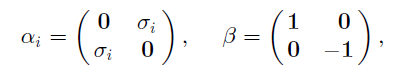
where 1 and 0 are the 2 × 2 unit matrix and null matrix, respectively, and the Pauli spin matrices σ_i are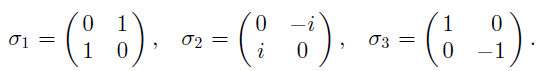

Check that these forms satisfy the anti commutation relations

[α_i, β] ≡ α_iβ + βα_i = 0 and [α_i, α_j] = 0, where i ≠ j = 1, 2, 3.

Equation 1.8