Standing waves (see Section 16. 6) can form when sound traveling inside a closed tube reflects from each end. These waves have a node at each (immovable) end. Standing waves can also form in a tube with open ends. In this case, the waves have antinodes rather than nodes at the ends. Use the requirement of antinodes at the tube ends to determine the relationship between wavelength and tube length for the fundamental and the next two harmonics for standing waves in a tube that is open at both ends.

Data From Section 16. 6

 

.......

Transcribed Image Text:

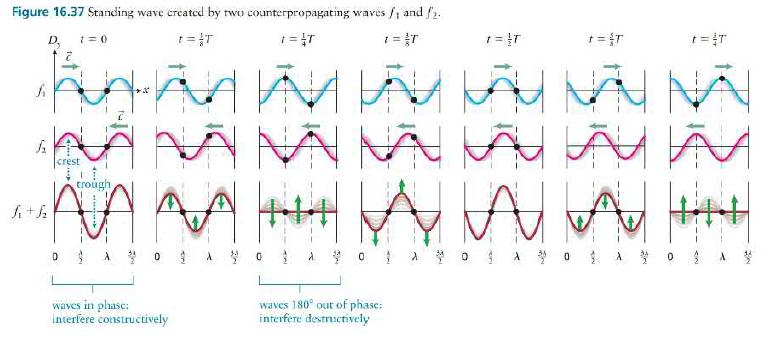
When a harmonic wave travels along a string that has a fixed end, the reflected wave interferes with the incident wave. Figure 16.36 shows the striking pattern that results from this interference. Initially the wave trav- els to the right, but as the reflected wave begins traveling back at tz, the two waves traveling in opposite directions create a pulsating stationary pattern. Some particles of the string-the nodes (see Section 16.3)-do not move at all, while the sinusoidal loops between the nodes move up and down rather than along the string. The motion has its greatest amplitude at points halfway between the nodes; these points are called antinodes. The pulsating stationary pattern caused by harmonic waves of the same amplitude traveling in opposite directions is called a standing wave. Figure 16.37 illustrates how standing waves come about. Each diagram shows a blue wave propagating to the right and a red wave propagating to the left. The two waves have the same amplitude and wavelength. The speed at which they propagate is determined by the medium and is there- fore the same for both waves. The bottom part of each diagram shows the sum of the two waves, obtained by adding, for each value of x, the vertical displacements of the two waves at that value of x. In the diagram labeled t = 0, the crests of the red wave have the same x coordinate as the crests of the blue wave, and the same is true for the troughs: Each trough in the red wave aligns with a trough in the blue wave. Waves like this, where comparable points align, are said to be in phase (their phases are the same). The two waves reinforce each other-they interfere constructively-and adding them yields a wave that has twice the amplitude. A quarter period later, at T, one has a crest when the other has a trough and the waves