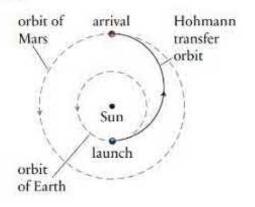
To travel between Earth and any other planet requires consideration of such things as expenditure of fuel energy and travel time. To simplify the calculations, one chooses a path such that the position in Earth's orbit where the launch occurs and the position in the other planet's orbit when the spacecraft arrives define a line that passes through the Sun, as shown for an Earth-Mars transfer in Figure P13.66. The path the spacecraft travels is an cllipse that has the Sun at one focus. Such a path is called a Hohmann transfer orbit, and the major axis of the ellipse, \(2 a\), is the sum of the radii of the Earth's and the other planet's orbits around the Sun. Take \(m_{\mathrm{S}}=1.99 \times 10^{30} \mathrm{~kg}, m_{\mathrm{M}}=6.42 \times 10^{23} \mathrm{~kg}\), \(m_{\mathrm{E}}=5.97 \times 10^{24} \mathrm{~kg}, a_{\mathrm{E}}=1.50 \times 10^{11} \mathrm{~m}\), and \(a_{\mathrm{M}}=\) \(2.28 \times 10^{11} \mathrm{~m}\), and assume the planets have circular orbits.

(a) What is the energy of the system comprising the Sun and a \(1000-\mathrm{kg}\) space probe in a Hohmann transfer orbit? 

\((b)\) What is the speed of the probe in this orbit, as a function of \(r\), the probe's radial distance from the Sun?

(c) What is the probe's speed relative to the Sun as the probe enters the transfer orbit?

(d) What is its speed relative to the Sun as it approaches Mars?

(e) Given that the orbital speed of Earth is about \(2.98 \times 10^{4} \mathrm{~m} / \mathrm{s}\), how much additional speed does the probe need to begin the transfer orbit? ( \(f\) ) What is the required launch speed from Earth's surface for a probe traveling to Mars in a Hohmann transfer orbit?

Data from Figure P13.66

Transcribed Image Text:

orbit of arrival Mars Hohmann transfer orbit orbit of Earth Sun launch