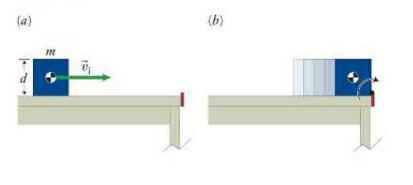
You shove a cube of inertia \(m\) and side length \(d\) so that it slides along a smooth table with speed \(v_{1}\) (Figure P12.77a). The cube then hits a raised lip at the end of the table. After it hits the lip, the cube begins to rotate about it (Figure 12.77b).

(a) Show that the magnitude of the cube's angular momentum about the lip before the collision is \(L=m d v_{i} / 2\).

(b) Explain why the angular momentum still has that value at the instant of collision, before the cube has had time to rotate much.

(c) What is the rotational acceleration of the cube the instant after it hits the lip?

(d) What maximum initial speed can the cube have so that it does not topple over the lip?

Data from Figure P12.27b

Transcribed Image Text:

(a) m (b)