Refer to Problem 55. Find the volume of a parallelepiped whose defining vectors are A = i

Question:

Refer to Problem 55. Find the volume of a parallelepiped whose defining vectors are A = i + 6k, B = 2i + 3j − 8k, and C = 8i − 5j + 6k.

Data from problem 55.

A parallelepiped is a prism whose faces are all parallelograms. Let A, B, and C be the vectors that define the parallelepiped shown in the figure. The volume V of the parallelepiped is given by the formula V = |(A × B)|· C|.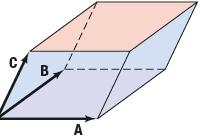

Find the volume of a parallelepiped if the defining vectors are A = 3i − 2j + 4k, B = 2i + j − 2k, and C = 3i − 6j − 2k.

Fantastic news! We've Found the answer you've been seeking!

Step by Step Answer:

Related Book For book-img-for-question

Precalculus Concepts Through Functions A Unit Circle Approach To Trigonometry

ISBN: 9780137945139

5th Edition

Authors: Michael Sullivan

See More Books

Question Posted: Jul 25, 2023 03:26 AM

See More Questions

Refer to Problem 55. Find the volume of a parallelepiped whose defining vectors are A = (1,0,6), B = (2,3,8) and C = (8,-5,6). Problem 55 A parallelepiped is a prism whose faces are all...
A parallelepiped is a prism whose faces are all parallelograms. Let A, B, and C be the vectors that define the parallelepiped shown in the figure. The volume V of the parallelepiped is given by the...
200 mL of an ideal gas is measured to have a mass of 19.57 grams at a temperature of 25 C and 1 atm pressure. What gas is this? D View hint for Question 48
A sales invoice included the following information: merchandise price, $12,000; terms 1/10, n/eom, FOB shipping point with prepaid freight of $900 added to the invoice. Assuming that a credit for...
Lamprino Appliance uses a perpetual inventory system. The following are three recent merchandising transactions: June 10 Purchased 10 televisions from Mitsu Industries on account. Invoice price, $300...
REQUIRED: Cost of production report under the following assumptions: Lost units - normal, discovered at the beginning Lost units - normal, discovered at the end Lost units - abnormal, discovered when...
Describe the role of strategic partnerships in app-based ride sharing technology business. LO.1
Gonzalvo Corporations balance sheet at the end of 2013 included the following items. The following information is available for 2014. 1. Treasury stock was purchased at a cost of $13,200. 2. Cash...
Sol Martinez Company's ending Inventory includes the following items. Cost per Market per Product Units Unit Unit Helmets $ 59 $ 55 Bats Shoes 200 Uniform 37 30 51 55 77 96 83 Compute the lower of...
In Problems 3962, identify and graph each polar equation. r = 30
In Problems 3962, identify and graph each polar equation. r = 3 + cos
Complete the table and use the result to estimate the limit. Use a graphing utility to graph the function to confirm your result. sin x lim x-0 X X f(x) -0.1 -0.01 -0.001 0 0.001 2 0.01 0.1
Because her insurance agency is in a lakeside community, Adriana always asks her homeowners clients what boating activities they engage in, and she is sure to add the Watercraft endorsement...
1. Among all assumptions in CVP analysis, which one do you think is the most critical? Explain. 2. How will you change the cost-volume-profit analysis if the assumption (you identify in the previous...
Construct a confidence interval for p-P2 at the given level of confidence. x =26, n =229, x2 = 31, n = 302, 95% confidence The researchers are % confident the difference between the two population...
AP 9-2 (Moving Expenses) In May of the current year, following a dispute with her immediate superior, Ms. Elaine Fox resigned from her job in Halifax and began to look for other employment. She was...
Minimize the number of states in the following DFA: A b b a a a b b b E B a a
Alice, a resident of Michigan, claims that Pet Food Company, Inc. put out some dog food that made her dog, Champ, sick. Champ is a prize winning poodle. He survived the pet food fiasco but only after...
Medi-Exam Health Services, Inc. (MEHS), located in a major metropolitan area, provides annual physical screening examinations, including a routine physical, EKG, and blood and urine tests. MEUS's...
Simplify the expression. -4 2
Simplify the expression. 4 -2
Simplify the expression. -4 -2
thumbs up if correct A stock paying no dividends is priced at $154. Over the next 3-months you expect the stock torpeither be up 10% or down 10%. The risk-free rate is 1% per annum compounded...
Question 17 2 pts Activities between affiliated entities, such as a company and its management, must be disclosed in the financial statements of a corporation as O significant relationships O segment...
Marchetti Company, a U.S.-based importer of wines and spirits, placed an order with a French supplier for 1,000 cases of wine at a price of 200 euros per case. The total purchase price is 200,000...

Refer to Problem 55. Find the volume of a parallelepiped whose defining vectors are A = i

Question:

B A

Step by Step Answer:

Precalculus Concepts Through Functions A Unit Circle Approach To Trigonometry

Students also viewed these Mathematics questions