A random process is composed of sample functions of the form where n(t) is a wide-sense stationary
Question:
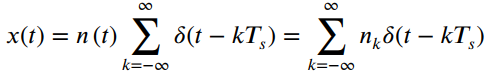
where n(t) is a wide-sense stationary random process with the auto correlation function Rn(Ï„), and nk = n(kTs).
(a) If Ts is chosen to satisfy
Rn(kTs) = 0, k = 1,2,..
so that the samples nk = n(kTs) are orthogonal, use Equation (7.35) to show that the power spectral density of x(t) is 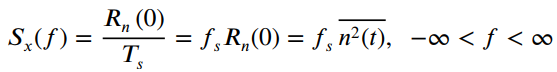
(b) If x(t) is passed through a filter with impulse response h(t) and frequency response function H(f), show that the power spectral density of the output random process, y(t), is 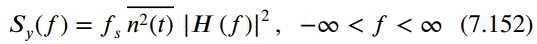
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Principles of Communications Systems, Modulation and Noise
ISBN: 978-8126556793
7th edition
Authors: Rodger E. Ziemer, William H. Tranter
Question Posted:





