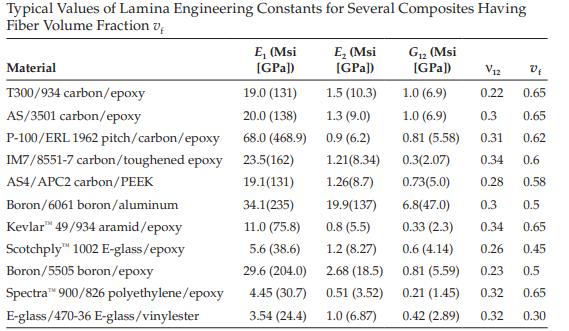
Determine the isotropic moduli \(\tilde{E}\) and \(\tilde{G}\) for a composite consisting of randomly oriented T300 CFs in a 934 epoxy matrix if the fibers are long enough to be considered continuous. Use the properties in Table 2.2. Compare the values of \(\tilde{E}\) and \(\tilde{G}\) calculated from the invariant expressions (Equations 6.43) with those calculated from the approximate expressions in Equations 6.44.

\(\begin{aligned}
& \tilde{E}=\frac{\left(U_1-U_4\right)\left(U_1+U_4\right)}{U_1} \\
& \tilde{G}=\frac{U_1-U_4}{2} \\
& \tilde{\mathrm{v}}=\frac{U_4}{U_1}
\end{aligned} \tag{6.43}\)

\(\tilde{E}=\frac{3}{8} E_1+\frac{5}{8} E_2, \quad \tilde{G}=\frac{1}{8} E_1+\frac{1}{4} E_2 \tag{6.44}\)

Table 2.2:

Transcribed Image Text:

Typical Values of Lamina Engineering Constants for Several Composites Having Fiber Volume Fraction v Material E (Msi [GPa]) E (Msi [GPa]) G (Msi [GPa]) V12 V T300/934 carbon/epoxy 19.0 (131) 1.5 (10.3) 1.0 (6.9) 0.22 0.65 AS/3501 carbon/epoxy 20.0 (138) 1.3 (9.0) 1.0 (6.9) 0.3 0.65 P-100/ERL 1962 pitch/carbon/epoxy 68.0 (468.9) 0.9 (6.2) 0.81 (5.58) 0.31 0.62 IM7/8551-7 carbon/toughened epoxy 23.5(162) 1.21(8.34) 0.3(2.07) 0.34 0.6 AS4/APC2 carbon/PEEK 19.1(131) 1.26(8.7) 0.73(5.0) 0.28 0.58 Boron/6061 boron/aluminum 34.1(235) 19.9(137) 6.8(47.0) 0.3 0.5 Kevlar 49/934 aramid/epoxy 11.0 (75.8) 0.8 (5.5) 0.33 (2.3) 0.34 0.65 Scotchply 1002 E-glass/epoxy 5.6 (38.6) 1.2 (8.27) 0.6 (4.14) 0.26 0.45 Boron/5505 boron/epoxy 29.6 (204.0) 2.68 (18.5) 0.81 (5.59) 0.23 0.5 Spectra 900/826 polyethylene/epoxy 4.45 (30.7) 0.51 (3.52) 0.21 (1.45) 0.32 0.65 E-glass/470-36 E-glass/vinylester 3.54 (24.4) 1.0 (6.87) 0.42 (2.89) 0.32 0.30