Repeat Problem 5.40, but consider the 2.5-mm thick stainless steel strip to emerge from the rolling mill
Question:
Repeat Problem 5.40, but consider the 2.5-mm thick stainless steel strip to emerge from the rolling mill with a speed of 35 m/s. With the temperature of the strip and the surrounding air, respectively, of 900°C and 30°C, determine the time-dependent rate of change of the strip temperature at a distance of 0.75 m and 1.5 m from the leading tip. Comment on your results and the estimated cooling rate compared to that in the previous problem.
Data from in Problem 5.40
In a sheet-metal rolling and stamping factory, a 2.5-mm thick strip of stainless steel is hot rolled, as is schematically depicted in the figure. The strip emerges from the furnace and the rolling mill with a temperature of 900°C with a speed of 10 m/s, and is exposed to atmospheric air at 30°C. Determine the time-dependent rate of change in the temperature of the strip at a distance of 0.75 m from the leading tip of the rolled steel strip. In modeling this problem, neglect all radiation heat transfer but consider lumped capacitance for conduction within the strip with convection heat transfer from both the top and bottom surfaces. Also, at what x-location from the leading tip is the minimum cooling rate and why? Where is the maximum cooling rate?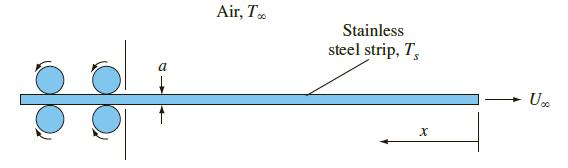
Step by Step Answer:

Principles Of Heat Transfer
ISBN: 9781305387102
8th Edition
Authors: Frank Kreith, Raj M. Manglik, Mark S. Bohn





