Consider the bivariate-normal Gibbs sampler of Example 15.4.

(i) Show that the marginal chain Xt has transition kernel

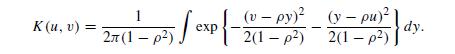
(ii) Show that the stationary distribution of the chain Xt is N(0, 1).
(iii) Show that Xt+1 = ρ2Xt + t , t = 1, 2, . . . , where t are i.i.d. with distribution N(0, 1−ρ4). Using this relation, show that Xt is a (scalar)
Markov chain with stationary distribution N(0, 1) (here you are not supposed to use the results of the previous parts, but rather to derive it directly).

Transcribed Image Text:

1 K(u, v) = = - 2x(1 - p) exp{-(-)-1} (v-py) (y-pu) | 2(1 p2) 2(1 p) | dy.