This exercise is to show that both sides of inequality (11.19) are sharp in that there are distributions F that are continuous and symmetric about zero for which the left- or right-side equalities are either attained or approached with arbitrary closeness.

(i) Let F has a rectangular distribution that is symmetric about zero. Show that ρw = 1; hence, the left side equality holds.

(ii) Let F have the pdf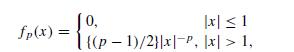

where p > 3. Verify that fp is a pdf. Then show that ρw, ρs → 0 as p → 3. Therefore, the right side of (11.19) can be approached with arbitrary closeness by choosing p sufficiently close to 3.

Transcribed Image Text:

0, x|1 fp(x) = {(p-1)/2}|x| P, |x| > 1,