14. (Tandem or Sequential Queueing System) In a computer store, customers arrive at a cashier desk at a Poisson rate of λ to pay for the goods they want to purchase. If the cashier is busy, then they wait in line until their turn on a first-come, first-served basis.

The time it takes for the cashier to serve a customer is exponential with mean 1/μ1, independent of the arrival process. After being served by the cashier, customers join a second queue and wait until their turn to receive the goods they have purchased. When a customer’s turn begins, it take an exponential period with mean 1/μ2 to be served, independent of the service times of other customers and service times and arrival times at the cashier’s desk. Clearly, the first station, cashier’s desk, is an M/M/1 queueing system. The departure process of the first station forms an arrival process for the second station, delivery desk. Show that the second station is also anM/M/1 queueing system and is independent of the queueing system at the first station.

Hint: For i ≥ 0, j ≥ 0, by the state (i, j) we mean that there are i customers in the first queueing system and j customers in the second one. Let π(i,j) be the long-run probability that there are i customers at the cashier’s desk and j customers at the delivery desk.Write down the set of balance equations for the entire process and show that

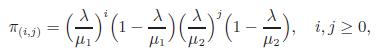
satisfy the balance equations. If this is shown, by Example 12.38, we have shown that π(i,j) is the product of the long-run probabilities that the first queueing system is M/M/1 and has i customers, and the second queueing system is M/M/1 and has j customers.

Transcribed Image Text:

(A)(-)(A)(-A) (i,j) i, j 0,