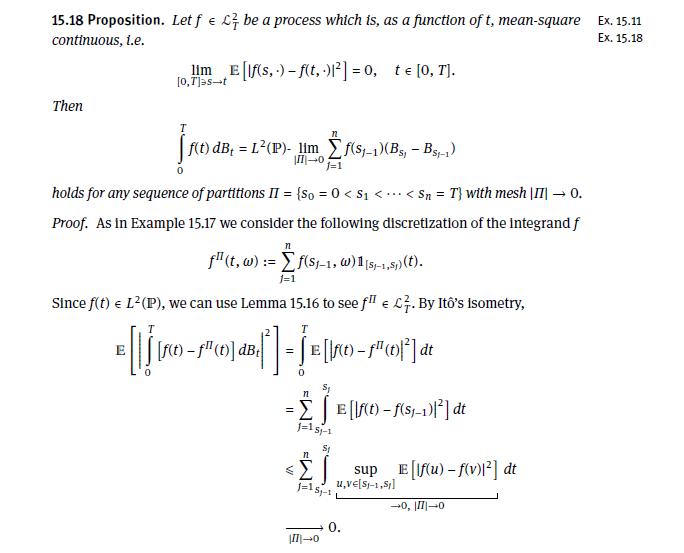
Adapt the proof of Proposition 15.18 and prove that we also have [lim _{|Pi| ightarrow 0}

Adapt the proof of Proposition 15.18 and prove that we also have \[\lim _{|\Pi| \rightarrow 0} \mathbb{E}\left[\sup _{t \leqslant T}\left|\int_{0}^{t} f(s) d B_{s}-\sum_{j=1}^{n} f\left(s_{j-1}\right)\left(B_{s_{j} \wedge t}-B_{s_{j-1} \wedge t}\right)\right|^{2}\right]=0\]

Data From Proposition 15.18

Transcribed Image Text:

15.18 Proposition. Let f = be a process which is, as a function of t, mean-square Ex. 15.11 continuous, i.e. lim E[f(s,-)-f(t,-)] = 0, t = [0, 1]. [0,T]s-t Ex. 15.18 Then n f(t) dB, L(P)- lim f(S-1) (Bs, - Bs-1) [[]] 0 1=1 holds for any sequence of partitions II = {So = 0 < S < < Sn = T} with mesh |II| 0. - Proof. As in Example 15.17 we consider the following discretization of the integrand f n f(t, w) = f(s-1, w) 15-1,s,)(t). 1=1 Since f(t) = L(P), we can use Lemma 15.16 to see f" e C. By It's isometry, [f(t) -f(t)] dB = n E[f(t)-f(S-1)] dt 1=1-51-1 St n sup E[f(u) - f(v)|] dt 1=15-1 L u,ve[S1-1,Sj] -0, 1-0 0. (f)0