At time \(t=0\), a parallel system \(S\) consisting of two elements \(e_{1}\) and \(e_{2}\) starts operating. Their lifetimes \(X_{1}\) and \(X_{2}\) are dependent with joint survival function

\[\bar{F}\left(x_{1}, x_{2}\right)=P\left(X_{1}>x_{1}, X_{2}>x_{2}\right)=\frac{1}{e^{+0.1 x_{1}}+e^{+0.2 x_{2}}-1}, \quad x_{1}, x_{2} \geq 0\]

(1) What are the distribution functions of \(X_{1}\) and \(X_{2}\) ?
(2) What is the probability that the system survives the interval \([0,10]\) ?
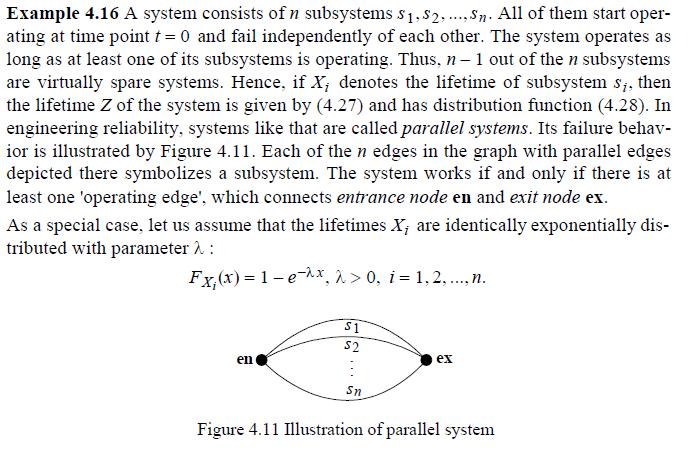
Note By definition, a parallel system is fully operating at a time point \(t\) if at least one of its elements is still operating at time \(t\), i.e., a parallel system fails at that time point when the last of its operating elements fails. See also example 4.16, page 176.

Data from Example 4.16

Example 4.16 A system consists of n subsystems S1, S2, ..., Sn. All of them start oper- ating at time point t = 0 and fail independently of each other. The system operates as long as at least one of its subsystems is operating. Thus, n-1 out of the n subsystems are virtually spare systems. Hence, if X, denotes the lifetime of subsystem si, then the lifetime Z of the system is given by (4.27) and has distribution function (4.28). In engineering reliability, systems like that are called parallel systems. Its failure behav- ior is illustrated by Figure 4.11. Each of the n edges in the graph with parallel edges depicted there symbolizes a subsystem. The system works if and only if there is at least one 'operating edge', which connects entrance node en and exit node ex. As a special case, let us assume that the lifetimes X; are identically exponentially dis- tributed with parameter : Fx(x)=1-ex, > 0, i = 1, 2,..., n. $1 52 ex en Sn Figure 4.11 Illustration of parallel system