Does Doeblins condition of Theorem 4 hold for the transition matrix (4.2.4)? Connect it with what was
Question:
Does Doeblin’s condition of Theorem 4 hold for the transition matrix (4.2.4)? Connect it with what was said in Example 4.2-3.
Example 4.2-3
Let a chain have one absorbing state, say,
where the stars ∗ represent positive numbers. The reader is invited to verify that in this case the solution to (4.2.2) is π = (0,0,1), that is, the limiting distribution is concentrated at the last state. It is not surprising at all, since with probability one the process will arrive at the absorbing state. For us, it is worth noting that Theorem 4 covers such cases.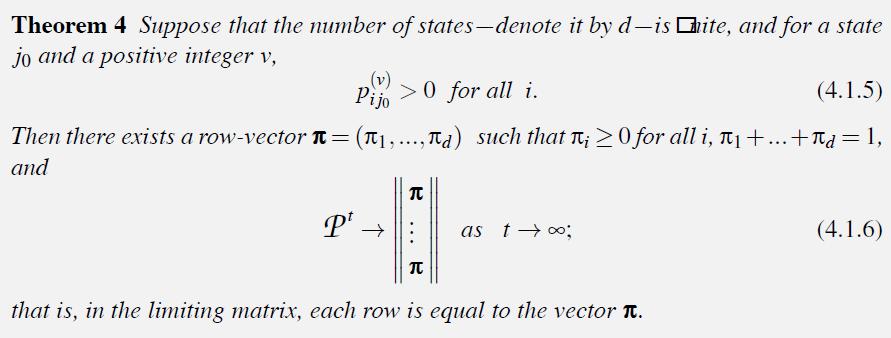
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





