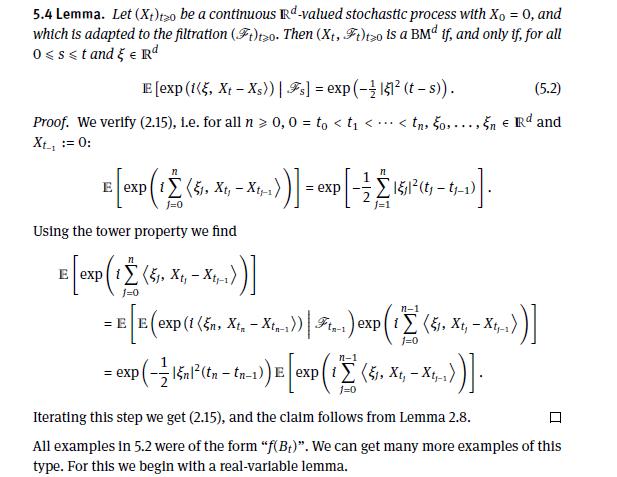
Let (left(X_{t}, mathscr{G}_{t} ight)) be an adapted, real-valued process with right continuous paths and finite left limits.

Let \(\left(X_{t}, \mathscr{G}_{t}\right)\) be an adapted, real-valued process with right continuous paths and finite left limits. Assume that \(\mathbb{P}\left(X_{t}-X_{s} \in A \mid \mathscr{G}_{S}\right)=\mathbb{P}\left(X_{t-s} \in A\right)\) holds for all \(0 \leqslant s)_{t \geqslant 0}\) enjoys the independent and stationary increment properties (B1), (B2).

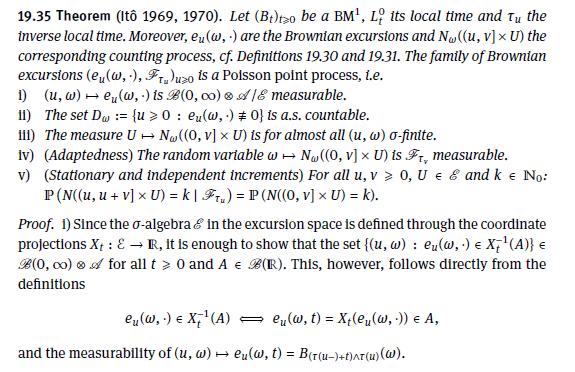
Mimic Lemma 5.4 Remark. This proves that Theorem 19.35.v) entails that the counting measure has stationary independent increments.

Data From Lemma 5.4

Data From Theorem 19.35

Transcribed Image Text:

5.4 Lemma. Let (X+) to be a continuous Rd-valued stochastic process with X = 0, and which is adapted to the filtration (F)to. Then (Xt, Ft)tzo is a BMd if, and only if, for all 0 < s < t and e Rd E [exp (1, Xt - Xs)) | Fs] = exp(-11 (ts)). (5.2) Proof. We verify (2.15), 1.e. for all n > 0,0 = to )] 1-0 = exp(-Kulfite-t-1) E exp (( X-X))]. -X-1 )] Iterating this step we get (2.15), and the claim follows from Lemma 2.8. All examples in 5.2 were of the form "f(B+)". We can get many more examples of this type. For this we begin with a real-variable lemma.