Let ({N(t), t geq 0}) be a nonhomogeneous Poisson process with trend function (Lambda(t)) and arrival time

Question:

Let $\{N(t), t \geq 0\}$ be a nonhomogeneous Poisson process with trend function $\Lambda(t)$ and arrival time point $T_{i}$ of the $i$ th Poisson event.

Given $N(t)=n$, show that the random vector $\left(T_{1}, T_{2}, \ldots, T_{n}\right)$ has the same probability distribution as $n$ ordered, independent and identically distributed random variables with distribution function

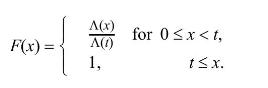
image text in transcribed