Tessa and Vanessa have agreed to meet at a caf between 16 and 17 o'clock. The arrival

Question:

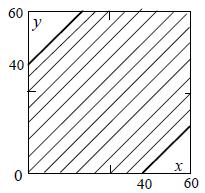
Tessa and Vanessa have agreed to meet at a café between 16 and 17 o'clock. The arrival times of Tessa and Vanessa are $X$ and $Y$, respectively. The random vector $(X, Y)$ is assumed to have a uniform distribution over the square

\[B=\{(x, y) ; 16 \leq x \leq 17,16 \leq y \leq 17\}\]

Who comes first will wait for 40 minutes and then leave.

What is the probability that Tessa and Vanessa will miss each other?

image text in transcribed

Fantastic news! We've Found the answer you've been seeking!

Step by Step Answer:

Related Book For book-img-for-question

Applied Probability And Stochastic Processes

ISBN: 9780367658496

2nd Edition

Authors: Frank Beichelt

See More Books

Question Posted: Apr 26, 2024 04:00 PM

See More Questions

The arrival times of students at the health center at a university is known to have a Poisson distribution with an average rate of 6 sick students arriving per hour. a. What is the expected number of...
Her Passion for Fashion Bobbi Jean Donnelly was hardened by her humble beginnings in rural Mississippi. The fourth of six children in a blue-collar working-class family, she spent the early years of...
Daniel Loureno is a Portuguese student in International Business at the University of Lisbon. Born in Reguengos de Monsaraz, he is an active sportsman, excelling in football and swimming. As a young...
(a) Compute the primary Class 1 NICs payable by the following weekly-paid employees for the week ending 14 August 2020: (i) Employee A has earnings for the week of 110. (ii) Employee B has earnings...
Your neighbor goes to the post office once a month and picks up two checks, one for $13,000 and one for $4,500. The larger check takes four days to clear after it is deposited; the smaller one takes...
The Sticky Company makes special glue that is used to make the layers of wood veneer adhere to make plywood. The glue-making process has been used for many years and the customers are satisfied with...
P 20-4 Governmental fund conversion worksheet The postclosing trial balance for the City of Fort Collins governmental funds at June 30, 2016, shows the following ledger account balances: DR CR Cash...
Roscoe contributes a personal use asset with an adjusted basis of $15,000 and a fair market value of $28,000 on the contribution date. Determine if any gain or loss is recognized and the basis under...
Jose Ruiz manages a car dealer's service department. His department is organized as a cost center. Costs for a recent quarter are shown below. Cost of parts Mechanics' wages Manager's salary Building...
Determine the mean length of a chord, which is randomly chosen in a circle with radius $r$. Consider separately the following ways how to randomly choose a chord: (1) For symmetry reasons, the...
Let the random vector $(X, Y)$ have a uniform distribution over a circle with radius $r=2$. Determine the distribution function of the point $(X, Y)$ from the center of this circle.
The time required for one complete cycle of a mass oscillating at the end of a spring is 0.25 s. What is the frequency of oscillation?
Solve the following linear system by Gaussian elimination with back-substitution without introducing fractions in your row-reduction. If there is no solution, explain why. -3x+8y + 82 = -8 -2x+ y -...
Introduction Some predictions are a slam dunk. Retail will continue to be driven by technology. Science fiction is coming to life in the form of robotics and virtual reality. And the Internet will...
Oswego Clay Pipe Company provides services of $ 5 0 , 0 0 0 to Southeast Water District # 4 5 on April 1 2 of the current year with terms 1 / 1 5 , n / 6 0 . What would Oswego record on April 1 2 ?...
Assume the following excerpts from a company's balance sheet: Property, plant, and equipment Beginning Balance $3,500,000 Ending Balance $3,700,000 $1,100,000 $800,000 Long-term investments During...
On January 1, 2021, Bonita Corp. had472,000shares of common stock outstanding. During 2021, it had the following transactions that affected the Common Stock account. February 1 Issued 125,000shares...
a. Prepare a frequency distribution table for lung and bronchus cancer rates for men. b. Construct the relative frequency and percentage distribution columns. c. Draw a histogram and polygon for the...
Which property determines whether a control is available to the user during run time? a. Available b. Enabled c. Unavailable d. Disabled
In 2019, Sunland Company had a break-even point of $388,000 based on a selling price of $5 per unit and fixed costs of $155,200. In 2020, the selling price and the variable costs per unit did not...
11. String Conversion Given a binary string consisting of characters '0's and '1', the following operation can be performed it: Choose two adjacent characters, and replace both the characters with...
Consider the table shown below to answer the question posed in part a. Parts b and c are independent of the given table. Callaway Golf (ELY) Alaska Air Group (ALK) Yum! Brands (YUM) Caterpillar...

Tessa and Vanessa have agreed to meet at a caf between 16 and 17 o'clock. The arrival

Question:

60 y 40 0 40 x 60

Step by Step Answer:

Hence ...View the full answer

Applied Probability And Stochastic Processes

Students also viewed these Business questions