The linear growth of the coefficients is essential for Corollary 21.31. a) Consider the case where (d=n=1,
Question:
The linear growth of the coefficients is essential for Corollary 21.31.
a) Consider the case where \(d=n=1, b(x)=-e^{x}\) and \(\sigma(x)=0\). Find the solution of this deterministic ODE and compare your findings for \(x \rightarrow+\infty\) with Corollary 21.31
b) Assume that \(|b(x)|+|\sigma(x)| \leqslant M\) for all \(x\). Find a simpler proof of Corollary 21.31. Hint. Find an estimate for \(\mathbb{P}\left(\left|\int_{0}^{t} \sigma\left(X_{s}^{x}\right) d B_{s}\right|>r\right)\).
c) Assume that \(|b(x)|+|\sigma(x)|\) grow, as \(|x| \rightarrow \infty\), like \(|x|^{1-\epsilon}\) for some \(\epsilon>0\). Show that one can still find a (relatively) simple proof of Corollary 21.31.
Data From Corollary 21.31

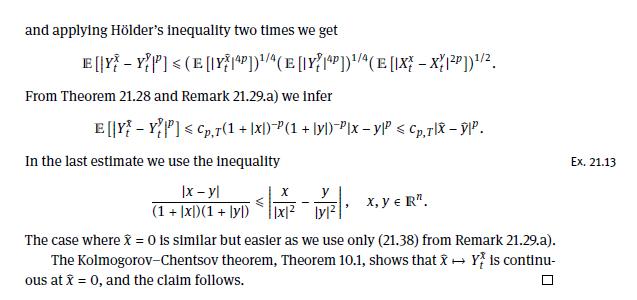
Step by Step Answer:

Brownian Motion A Guide To Random Processes And Stochastic Calculus De Gruyter Textbook
ISBN: 9783110741254
3rd Edition
Authors: René L. Schilling, Björn Böttcher





