In exercise 6 , if the states \(|1angle,|2angle,|3angle\) are eigenstates of the Hamiltonian \(\hat{H}\), write the evolution in time of the density matrix \(\hat{ho}\).

Data From Exercise 6:-

Consider the density matrix

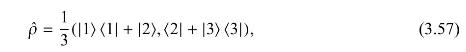
where \(|1angle\) and \(|2angle \pm|3angle\) are eigenstates of some operator \(\hat{A}\). Calculate the average value of \(A\).

Transcribed Image Text:

= (11) (1| + |2), (2| + |3) (31), (3.57)