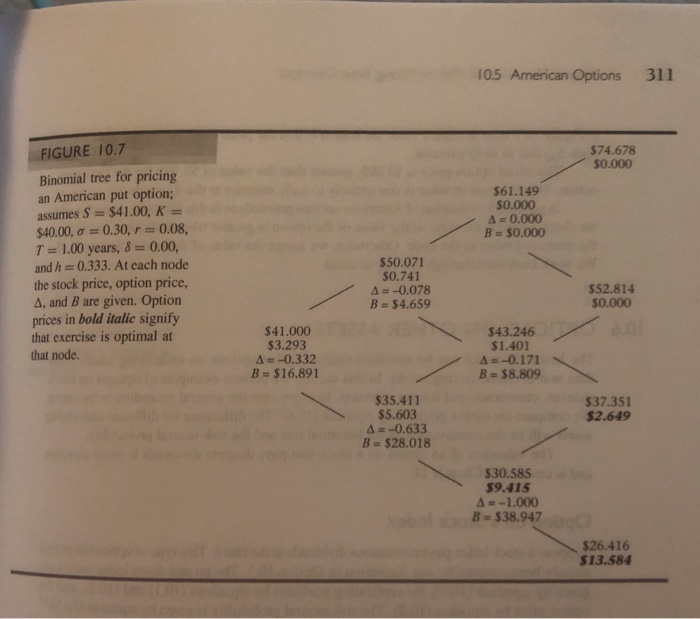
1. (10 pts.) Consider a 9-month dollar-denominated European put option on British pounds. You are given: (6) The current exchange rate is 1.43 US dollars per pound. (ii) The strike price of the put is 1.56 US dollars per pound. (ii) The volatility of the exchange rate is 0.3. (iv) The US dollar continuously compounded risk-free interest rate is 8%. (v) The British pound continuously compounded risk-free interest rate is 9%. Using a three-period binomial tree, calculate the price of the put option (show A, B, and option price on the tree as Figure 10.7 on page 311 in the textbook) 105 American Options 311 FIGURE I0.7 $74.678 50.000 Binomial tree for pricing an American put option; assumes S- $41.00, K $40.00, -: 0.30, r 0.08, 1.00 years, = 0.00, and h = 0.333. At each node the stock price, option price, A, and B are given. Option prices in bold italic signify that exercise is optimal at that node. $61.149 $0.000 0.000 B $0.000 $50.071 50.741 =-0.078 $4.659 8 $52.814 $0.000 $41.000 $3.293 $43.246 $1.401 A--0.171 $8.809 -0.332 $16.891 $35.411 $5.603 A-0.633 B-$28.018 $37.351 $2.649 30.585 $9.415 =-1.000 B-$38.947 $26.416 $13.584 1. (10 pts.) Consider a 9-month dollar-denominated European put option on British pounds. You are given: (6) The current exchange rate is 1.43 US dollars per pound. (ii) The strike price of the put is 1.56 US dollars per pound. (ii) The volatility of the exchange rate is 0.3. (iv) The US dollar continuously compounded risk-free interest rate is 8%. (v) The British pound continuously compounded risk-free interest rate is 9%. Using a three-period binomial tree, calculate the price of the put option (show A, B, and option price on the tree as Figure 10.7 on page 311 in the textbook) 105 American Options 311 FIGURE I0.7 $74.678 50.000 Binomial tree for pricing an American put option; assumes S- $41.00, K $40.00, -: 0.30, r 0.08, 1.00 years, = 0.00, and h = 0.333. At each node the stock price, option price, A, and B are given. Option prices in bold italic signify that exercise is optimal at that node. $61.149 $0.000 0.000 B $0.000 $50.071 50.741 =-0.078 $4.659 8 $52.814 $0.000 $41.000 $3.293 $43.246 $1.401 A--0.171 $8.809 -0.332 $16.891 $35.411 $5.603 A-0.633 B-$28.018 $37.351 $2.649 30.585 $9.415 =-1.000 B-$38.947 $26.416 $13.584