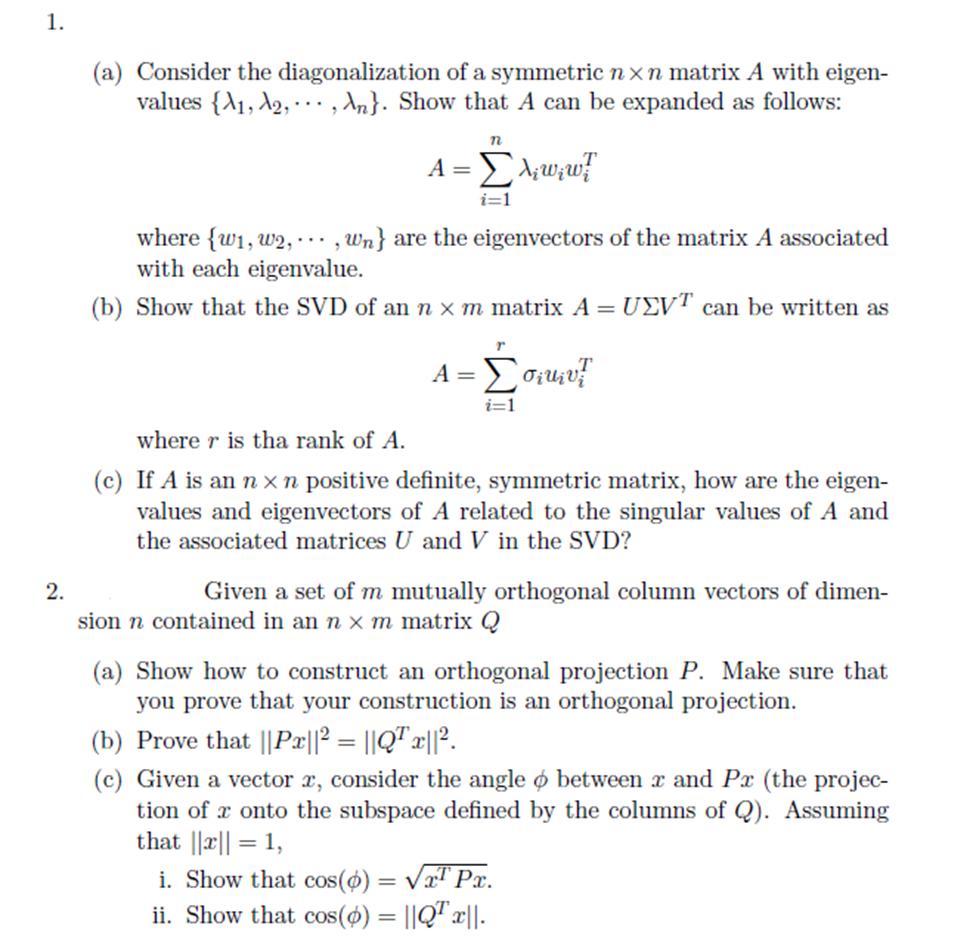
1. 2. (a) Consider the diagonalization of a symmetric nxn matrix A with eigen- values {, A2, An). Show that A can be expanded as follows: . n A = [Xww! i=1 where {w, W2, , wn} are the eigenvectors of the matrix A associated with each eigenvalue. (b) Show that the SVD of an nx m matrix A = UEVT can be written as r A = 0Uv! i=1 where r is tha rank of A. (c) If A is an n x n positive definite, symmetric matrix, how are the eigen- values and eigenvectors of A related to the singular values of A and the associated matrices U and V in the SVD? Given a set of m mutually orthogonal column vectors of dimen- sion n contained in an n x m matrix Q (a) Show how to construct an orthogonal projection P. Make sure that you prove that your construction is an orthogonal projection. (b) Prove that ||Pr|| = ||Qx||. (c) Given a vector a, consider the angle between x and Pr (the projec- tion of x onto the subspace defined by the columns of Q). Assuming that ||*|| = 1, i. Show that cos(o)=xT Px. ii. Show that cos(p) = ||QT||.