1. (20 Points) Let X be a random variable with PDF f(x) = J c(1 - x2), -1

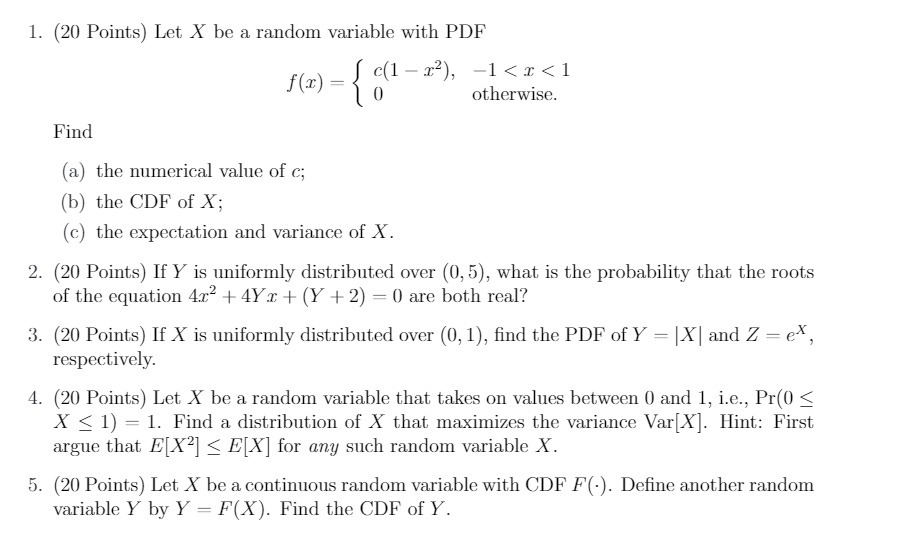
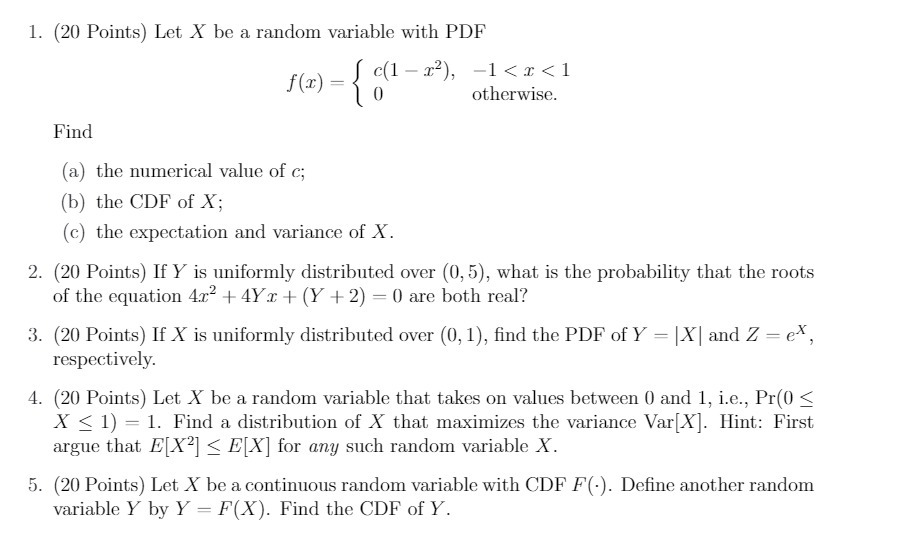
1. (20 Points) Let X be a random variable with PDF c(l F), 1 < x < 1 {0 Find (a) the numerical value of c; (b) the CDF of X; (c) the expectation and variance of X. otherwise. 2. (20 Points) If Y is uniformly distributed over (O, 5), what is the probability that the roots of the equation 4x2 + 4Y:r + (Y + 2) O are both real? 3. (20 Points) If X is uniformly distributed over (O, 1), find the PDF of Y IXI and Z ex, respectively. 4. (20 Points) Let X be a random variable that takes on values between O and 1, i.e., < 1. Find a distribution of X that maximizes the variance Var[X]. Ilint: First argue that ECX2] < E[X] for any such random variable X. 5. (20 Points) Let X be a continuous random variable with CDF F(). Define another random variable Y by Y F(X). Find the CDF of Y.