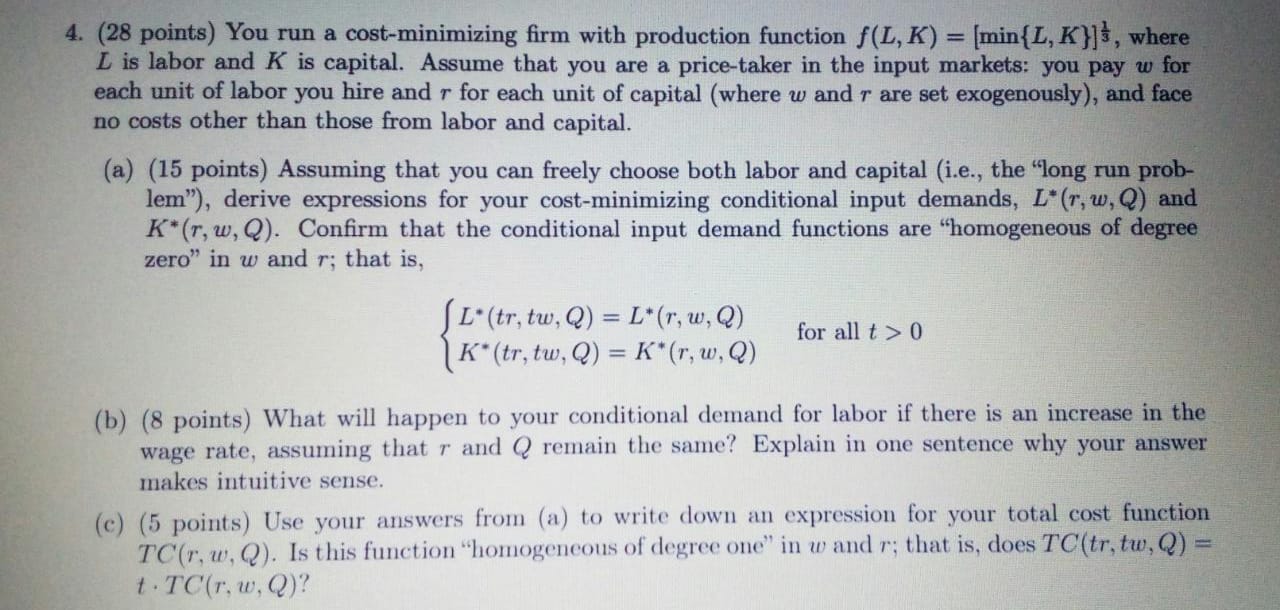
1. (27 points) For each of the following production functions, sketch a representative isoquant (2 points). Calculate the marginal product for each input, and indicate whether each marginal product is dimin- ishing, constant, or increasing (3 points). Calculate the marginal rate of technical substitution for each function (2 points). Also indicate whether the function exhibits constant, increasing, or diminishing returns to scale (2 points). (a) F(L, K) = LK3 ( b ) F ( L, K ) = L +3k (c) F(L, K) = (min { L, K } )2. (21 points) Consider the production function f( L, K) = 214Ki. (a) (15 points) Find the associated (long run) total, average, and marginal cost curves. (b) (6 points) Sketch the total, average, and marginal cost curves.4. (28 points) You run a cost-minimizing firm with production function f(L, K) = [min {L, K} ]s, where L is labor and K is capital. Assume that you are a price-taker in the input markets: you pay w for each unit of labor you hire and r for each unit of capital (where w and r are set exogenously), and face no costs other than those from labor and capital. (a) (15 points) Assuming that you can freely choose both labor and capital (i.e., the "long run prob- lem"), derive expressions for your cost-minimizing conditional input demands, L' (r, w, Q) and K*(r, w, Q). Confirm that the conditional input demand functions are "homogeneous of degree zero" in w and r; that is, J L. (tr, tw, Q) = L*(r, w, Q) K* (tr, tw, Q) = K* (r, w, Q) for all t > 0 (b) (8 points) What will happen to your conditional demand for labor if there is an increase in the wage rate, assuming that r and Q remain the same? Explain in one sentence why your answer makes intuitive sense. (c) (5 points) Use your answers from (a) to write down an expression for your total cost function TC(r, w, Q). Is this function "homogeneous of degree one" in w and r; that is, does TC(tr, tw, Q) = t . TC(r, w, Q)?1. (24 points) You manage a factory that produces cans of peanut butter. The current market price is $10/can, and you know the following about your costs: MC(5) = 10, ATC(5) = 6 MC(4) = 4, ATC(4) = 4 (a) (8 points) A case of food poisoning breaks out due to your peanut butter, and you lose a lawsuit against your company. As punishment, Judge Judy decides to take away all of your profits, and considers the following two options to be equivalent: i. Pay a lump sum in the amount of your profits. ii. Impose a tax of $[P - ATC(q* )] per can, since that is your current profit per can, where q' is the profit maximizing output before the lawsuit. Explain briefly why she is right or wrong. (b) (8 points) Judge Judy gives you the option of choosing either plan. Which plan would you choose? Provide intuition. Hint: a clear diagram may be helpful. (c) (8 points) In the following year, you finally pay off the court ordered amount and everything returns to normal. However, an employee tells you that at current levels of production, actual costs are double what you initially thought. After carefully looking at the cost information, you decide to continue producing the exact same amount. Could you still be maximizing profits?2. (32 points) Some questions about short-term and long-term costs: (a) (8 points) What is the relationship between the long-run average cost curve and the short-run average cost curve? Please show graphically. (b) (8 points) What algebraic condition describes a firm that is at an output level that maximizes its profits, given its capital in the short-term? (c) (8 points) What two algebraic conditions describe a firm that is at a capital level that minimizes its costs in the long-term? (d) (8 points) If a firm is characterized by short-run marginal cost that is greater than long-run marginal cost and short-run average cost greater than long-run average cost, how should it change its capital level in the long-run to minimize costs?3. (6 points) Suppose, in the short run, the output of widgets is supplied by 100 identical competitive firms, each having a cost function: c. ( y ) - 543 + 2 The demand for widgets is given by: y"(p) = 6400/p (a) (3 points) Obtain the short run industry supply function for widgets. (b) (3 points) Obtain the short run equilibrium price of widgets, and the output of widgets supplied by each firm.4. (22 points) Sebastian owns a coffee factory in Argentina. His production function is: F(K, L) = (K - 1)+14 Consider the cost of capital to be r and the wage to be w. Both inputs are variable, and Sebastian faces no fixed costs. (a) (2 points) What is the MRTS of labor for capital? (b) (5 points) What are Sebastian's input demands, conditional on the quantity (q) he wants to produce? [Hint: Treat w and r as parameters.] (c) (3 points) Show that Sebastian's long run cost function is C(q) = r + 2(wr) 2q2. (d) (5 points) What is the supply function of Sebastian's firm? Consider now that r = 4, w = 1, and that the market demand for coffee is Q = 20 - P. There are 7 other companies operating in this market, all with cost structures identical to Sebastian's company. (e) (6 points) What is the aggregate supply in this market? (f) (6 points) Calculate the equilibrium price, aggregate quantity sold, quantity sold by each firm, and economic profit of each firm. (g) (5 points) Can this be a long run equilibrium? Why? How will the supply side of the market adjust in the long run? (h) (6 points) What is going to be the price in the long run? How many firms will be present in this market in the long run? How much will each firm produce