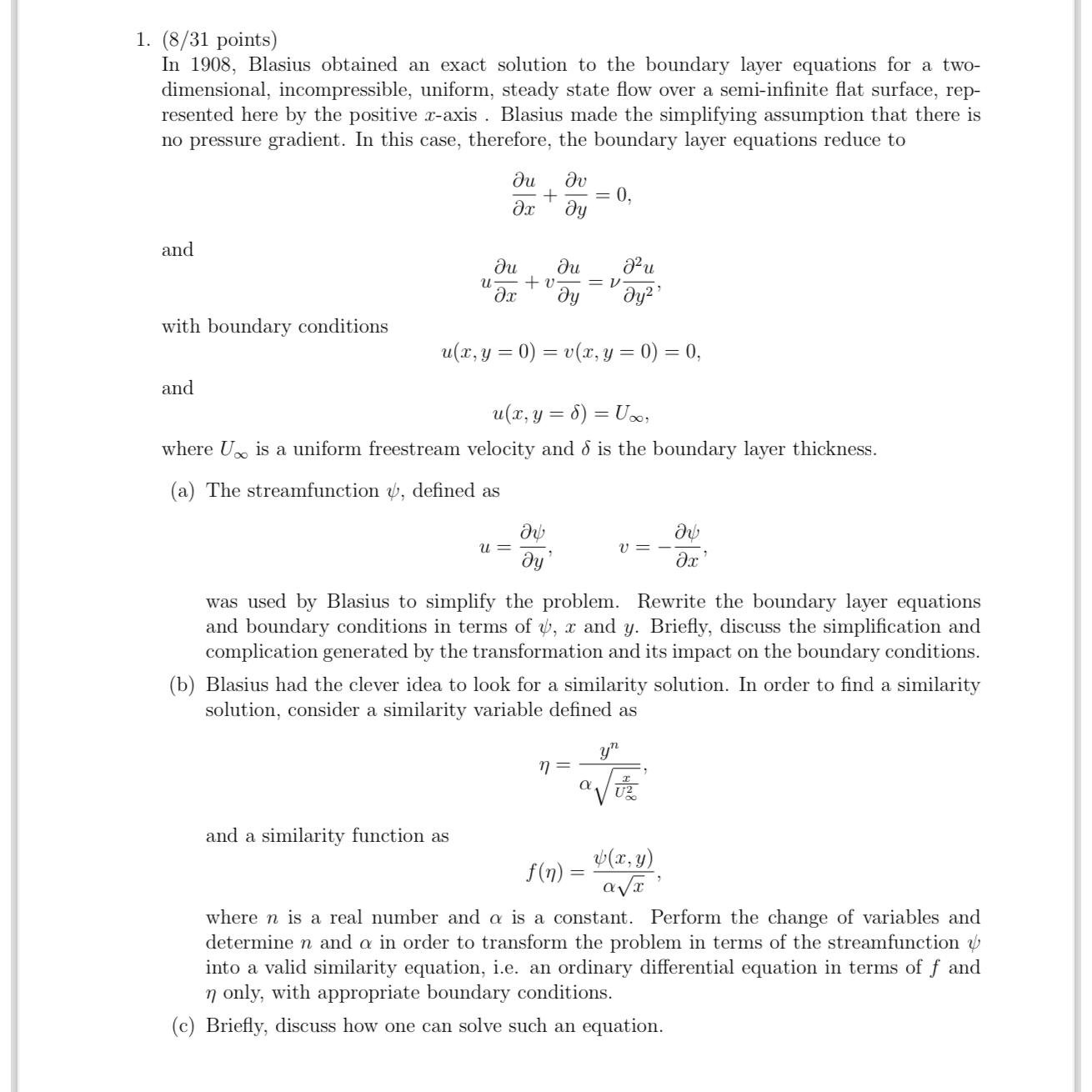
1. (8/31 points) In 1908, Blasius obtained an exact solution to the boundary layer equations for a two- dimensional, incompressible, uniform, steady state flow over a semi-infinite flat surface, rep- resented here by the positive x-axis. Blasius made the simplifying assumption that there is no pressure gradient. In this case, therefore, the boundary layer equations reduce to and with boundary conditions and + 0, Ju + v =V Jy' u(x, y = 0) = v(x, y = 0) = 0, u(x, y = 8) = U, where U is a uniform freestream velocity and 8 is the boundary layer thickness. (a) The streamfunction, defined as u = Jy' v = = was used by Blasius to simplify the problem. Rewrite the boundary layer equations and boundary conditions in terms of , x and y. Briefly, discuss the simplification and complication generated by the transformation and its impact on the boundary conditions. (b) Blasius had the clever idea to look for a similarity solution. In order to find a similarity solution, consider a similarity variable defined as yn n = a U2 and a similarity function as f(n) = v(x, y) where n is a real number and a is a constant. Perform the change of variables and determine n and a in order to transform the problem in terms of the streamfunction into a valid similarity equation, i.e. an ordinary differential equation in terms of f and n only, with appropriate boundary conditions. (c) Briefly, discuss how one can solve such an equation.