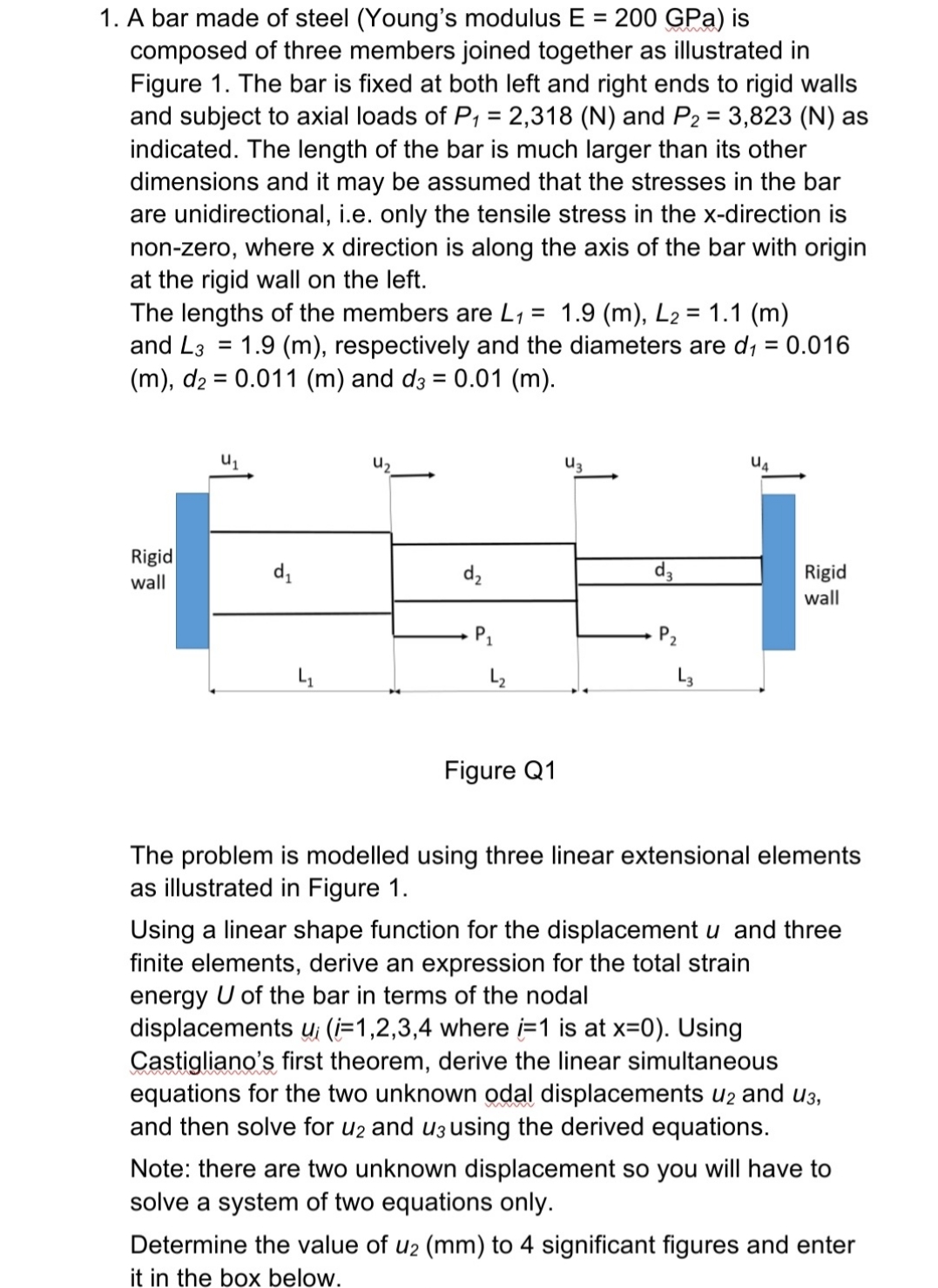
1. A bar made of steel (Young's modulus E = 200 GPa) is composed of three members joined together as illustrated in Figure 1. The bar is fixed at both left and right ends to rigid walls and subject to axial loads of P = 2,318 (N) and P2 = 3,823 (N) as indicated. The length of the bar is much larger than its other dimensions and it may be assumed that the stresses in the bar are unidirectional, i.e. only the tensile stress in the x-direction is non-zero, where x direction is along the axis of the bar with origin at the rigid wall on the left. The lengths of the members are L = 1.9 (m), L2 = 1.1 (m) = and L3 1.9 (m), respectively and the diameters are d = 0.016 (m), d2 0.011 (m) and d3 = 0.01 (m). = Rigid wall U u U3 44 d d Rigid wall P1 P2 L , L3 Figure Q1 The problem is modelled using three linear extensional elements as illustrated in Figure 1. Using a linear shape function for the displacement u and three finite elements, derive an expression for the total strain energy U of the bar in terms of the nodal displacements U (i=1,2,3,4 where i=1 is at x=0). Using Castigliano's first theorem, derive the linear simultaneous equations for the two unknown odal displacements U2 and 43, and then solve for u2 and 43 using the derived equations. Note: there are two unknown displacement so you will have to solve a system of two equations only. Determine the value of 42 (mm) to 4 significant figures and enter it in the box below.