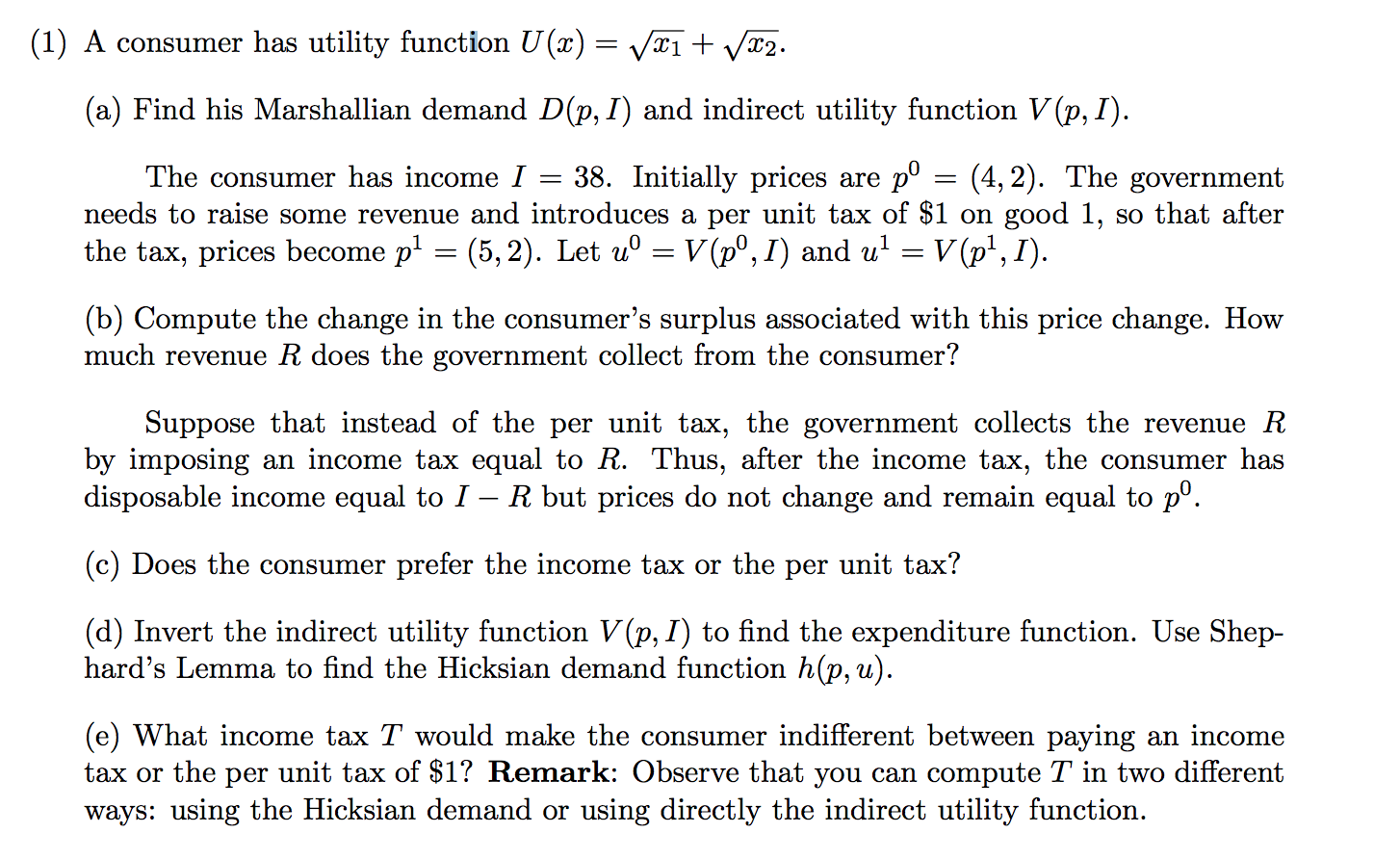
(1) A consumer has utility function U(x) = 1 + 2. (a) Find his Marshallian demand D(p, I) and indirect utility function V (p, I). The consumer has income I = 38. Initially prices are p = (4,2). The government needs to raise some revenue and introduces a per unit tax of $1 on good 1, so that after the tax, prices become p = (5,2). Let u = V (p, I) and u = V (p, I). (b) Compute the change in the consumer's surplus associated with this price change. How much revenue R does the government collect from the consumer? Suppose that instead of the per unit tax, the government collects the revenue R by imposing an income tax equal to R. Thus, after the income tax, the consumer has disposable income equal to I- R but prices do not change and remain equal to p. (c) Does the consumer prefer the income tax or the per unit tax? (d) Invert the indirect utility function V(p, I) to find the expenditure function. Use Shep- hard's Lemma to find the Hicksian demand function h(p, u). (e) What income tax T would make the consumer indifferent between paying an income tax or the per unit tax of $1? Remark: Observe that you can compute T in two different ways: using the Hicksian demand or using directly the indirect utility function.