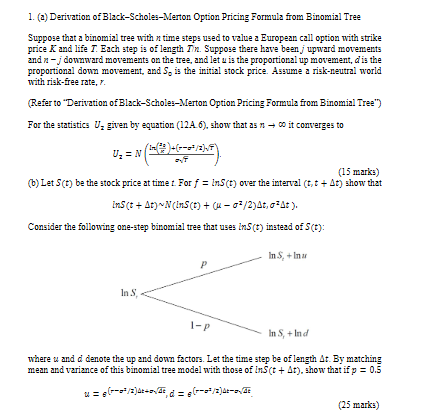
1. (a) Derivation of Black-Scholes-Merton Option Pricing Formula from Binomial Tree Suppose that a binomial tree with time steps used to value a European call option with strike price K and life T. Each step is of length Tin. Suppose there have been j upward movements and n-j downward movements on the tree, and let u is the proportional up movement, d'is the proportional down movement, and S, is the initial stock price. Assume a risk-neutral world with risk-free rate, 7. (Refer to "Derivation of Black-Scholes-Merton Option Pricing Formula from Binomial Tree") For the statistics U given by equation (12A.6), show that as it converges to ~= ( ) + ( ~~ / 2]}~/F) U = N + (15 marks) (b) Let S (t) be the stock price at timet. For f = InS(t) over the interval (t, t + At) show that InS (t + At)~N(InS(t) + (x - 0/2) 4t, oat), Consider the following one-step binomial tree that uses Ins(t) instead of S(t): In S, +Ina In S, 1-P In S, + Ind where u and d denote the up and down factors. Let the time step be of length At. By matching mean and variance of this binomial tree model with those of inS(t + At), show that if p = 0.5 x = e(r=0/2]4t+0x/4, d = g(---/2)4sode (25 marks)