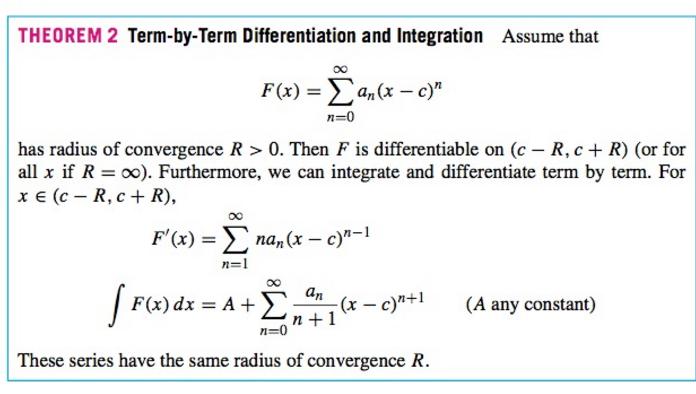
1 . a) Find the Taylor series for itself.] (1-2)2 [Hint: take the derivative of don't multiply by b) Apply Theorem 2 in section 10.6 to determine the interval of convergence of the above series. 1 2 3 c) The series s++ + converges, by the ratio test. What does it converge to? [Hint: this 4 8 16 is still part of the same problem.] 1 d) Ask a calculator, or WolframAlpha, for the decimal expansion of Explain why. It has a cool pattern. 9801 THEOREM 2 Term-by-Term Differentiation and Integration Assume that antx F(x)=an(x- n=0 has radius of convergence R > 0. Then F is differentiable on (c - R, c + R) (or for all x if R = 00). Furthermore, we can integrate and differentiate term by term. For xe (c - R,c+R), F(x)=nan(x- c)"-1 n=1 F(x) dx : 8 an F(x) dx = A + -(x - c)"+1 (A any constant) (n+1 =0 These series have the same radius of convergence R.