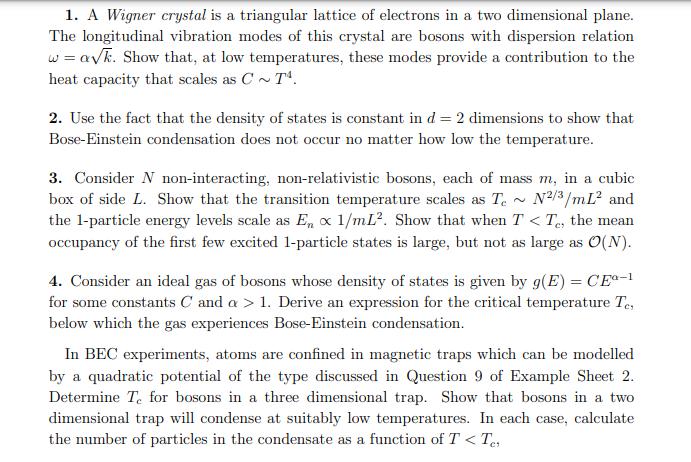
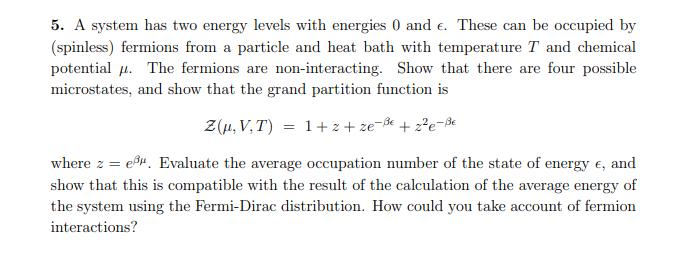
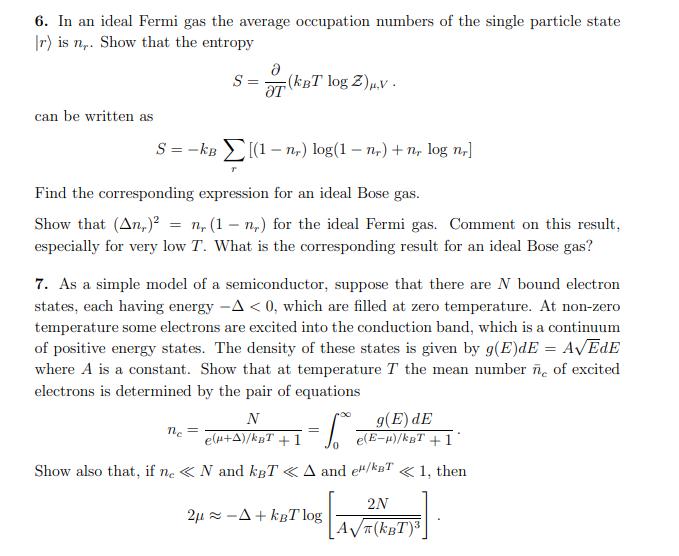
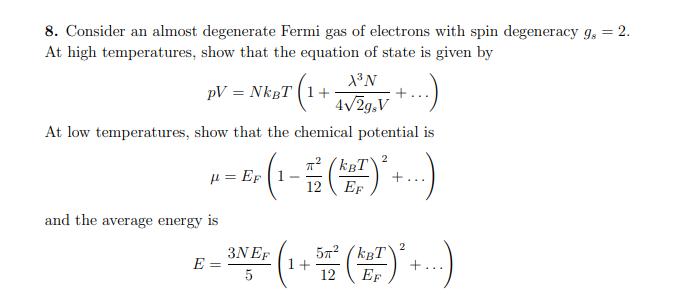
1. A Wigner crystal is a triangular lattice of electrons in a two dimensional plane. The longitudinal vibration modes of this crystal are bosons with dispersion relation w = ak. Show that, at low temperatures, these modes provide a contribution to the heat capacity that scales as CT. 2. Use the fact that the density of states is constant in d = 2 dimensions to show that Bose-Einstein condensation does not occur no matter how low the temperature. 3. Consider N non-interacting, non-relativistic bosons, each of mass m, in a cubic box of side L. Show that the transition temperature scales as TN2/3/mL and the 1-particle energy levels scale as E x 1/mL. Show that when T < Te, the mean occupancy of the first few excited 1-particle states is large, but not as large as O(N). 4. Consider an ideal gas of bosons whose density of states is given by g(E) = CE-1 for some constants C and a > 1. Derive an expression for the critical temperature Tes below which the gas experiences Bose-Einstein condensation. In BEC experiments, atoms are confined in magnetic traps which can be modelled by a quadratic potential of the type discussed in Question 9 of Example Sheet 2. Determine Te for bosons in a three dimensional trap. Show that bosons in a two dimensional trap will condense at suitably low temperatures. In each case, calculate the number of particles in the condensate as a function of T < Te 5. A system has two energy levels with energies 0 and e. These can be occupied by (spinless) fermions from a particle and heat bath with temperature T and chemical potential. The fermions are non-interacting. Show that there are four possible microstates, and show that the grand partition function is Z(, V.T) = 1+ 2+ zeBe +2e-Be where z = e. Evaluate the average occupation number of the state of energy , and show that this is compatible with the result of the calculation of the average energy of the system using the Fermi-Dirac distribution. How could you take account of fermion interactions? 6. In an ideal Fermi gas the average occupation numbers of the single particle state [r) is n.. Show that the entropy can be written as S = T S = -kB [(1 - n,) log(1 n) + n, log n,] (KBT log Z).V. Find the corresponding expression for an ideal Bose gas. = Show that (An,) n, (1 n,) for the ideal Fermi gas. Comment on this result, especially for very low T. What is the corresponding result for an ideal Bose gas? - nc 7. As a simple model of a semiconductor, suppose that there are N bound electron states, each having energy -A